灰度共生矩阵（GLCM）并计算能量、熵、惯性矩、相关性（matlab）（待总结）

关于灰度共生矩阵的介绍可参考

http://blog.csdn.net/chuminnan2010/article/details/22035751

http://blog.csdn.net/xuezhisd/article/details/8908824

http://blog.csdn.net/xuexiang0704/article/details/8713204

http://cn.mathworks.com/help/images/ref/imlincomb.html?refresh=true

理论介绍

http://blog.csdn.net/lskyne/article/details/8659225
http://blog.csdn.net/light_lj/article/details/26098815
http://blog.csdn.net/kezunhai/article/details/42001477

共生矩阵的物理意义
http://blog.csdn.net/light_lj/article/details/26098815

下面给出不同的NumLevels的例子

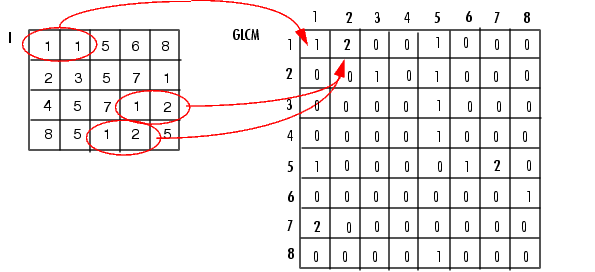
gray = [1 1 5 6 8  2 3 5 7 1  4 5 7 1 2  8 5 1 2 5];
GLCM = graycomatrix(gray,'GrayLimits',[])GLCM =1     2     0     0     1     0     0     00     0     1     0     1     0     0     00     0     0     0     1     0     0     00     0     0     0     1     0     0     01     0     0     0     0     1     2     00     0     0     0     0     0     0     12     0     0     0     0     0     0     00     0     0     0     1     0     0     0GLCM = graycomatrix(gray, 'GrayLimits',[],'offset', [0 1])GLCM =1     2     0     0     1     0     0     00     0     1     0     1     0     0     00     0     0     0     1     0     0     00     0     0     0     1     0     0     01     0     0     0     0     1     2     00     0     0     0     0     0     0     12     0     0     0     0     0     0     00     0     0     0     1     0     0     0GLCM = graycomatrix(gray, 'GrayLimits',[],'offset', [0 1],'NumLevels',8)GLCM =1     2     0     0     1     0     0     00     0     1     0     1     0     0     00     0     0     0     1     0     0     00     0     0     0     1     0     0     01     0     0     0     0     1     2     00     0     0     0     0     0     0     12     0     0     0     0     0     0     00     0     0     0     1     0     0     0

这里写图片描述

上图显示了如何求解灰度共生矩阵，以（1，1）点为例，GLCM（1，1）值为1说明只有一对灰度为1的像素水平相邻。GLCM（1，2）值为2，是因为有两对灰度为1和2的像素水平相邻。（1,5）出现一次，所以在（1.5）位置上标记1，没出现（1,6)所以为0；

上面所有的参数都是默认设置，NumLevels=8， 下面考虑NumLevels=3 的情况

gray = [1 1 5 6 8  2 3 5 7 1  4 5 7 1 2  8 5 1 2 5];GL(2) = max(max(gray));
GL(1) = min(min(gray));
if GL(2) == GL(1)SI = ones(size(gray));
else slope = NumLevels/(GL(2) - GL(1));intercept = 1 - (slope*(GL(1)));SI = floor(imlincomb(slope,gray,intercept,'double'));
end
%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%
SI =1     1     2     3     41     1     2     3     12     2     3     1     14     2     1     1     2
%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%SI(SI > NumLevels) = NumLevels;
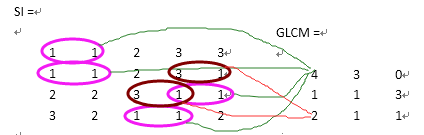
SI(SI < 1) = 1;SI =1     1     2     3     31     1     2     3     12     2     3     1     13     2     1     1     2

上面给出了如何将初始矩阵gray变成3阶的灰度级，SI就是gray的3阶灰度级矩阵。

这里写图片描述

上图显示了如何求解3级灰度共生矩阵，以（1，1）点为例，GLCM（1，1）值为4说明只有4对灰度为1的像素水平相邻。GLCM（3，1）值为2，是因为有两对灰度为3和1的像素水平相邻。（2,1）出现一次，所以在（2.1）位置上标记1，没出现（1,3)所以为0；

gray = [1 1 5 6 8  2 3 5 7 1  4 5 7 1 2  8 5 1 2 5];
[GLCM,SI] = graycomatrix(gray,'NumLevels',3,'G',[])GLCM =4     3     01     1     32     1     1SI =1     1     2     3     31     1     2     3     12     2     3     1     13     2     1     1     2

用3阶灰度级去计算四个共生矩阵P,取距离为1，角度分别为0,45,90,135 

gray = [1 1 5 6 82 3 5 7 14 5 7 1 28 5 1 2 5];
offsets = [0 1;-1 1;-1 0;-1 -1];
m = 3; % 3阶灰度级
[GLCMS,SI] = graycomatrix(gray,'GrayLimits',[],'Of',offsets,'NumLevels',m);
P = GLCMS;
[kk,ll,mm] = size(P);
% 对共生矩阵归一化
%---------------------------------------------------------
for n = 1:mmP(:,:,n) = P(:,:,n)/sum(sum(P(:,:,n)));
end%-----------------------------------------------------------
%对共生矩阵计算能量、熵、惯性矩、相关4个纹理参数
%-----------------------------------------------------------
H = zeros(1,mm);
I = H;
Ux = H;      Uy = H;
deltaX= H;  deltaY = H;
C =H;
for n = 1:mmE(n) = sum(sum(P(:,:,n).^2)); %能量for i = 1:kkfor j = 1:llif P(i,j,n)~=0H(n) = -P(i,j,n)*log(P(i,j,n))+H(n); %熵endI(n) = (i-j)^2*P(i,j,n)+I(n);  %惯性矩Ux(n) = i*P(i,j,n)+Ux(n); %相关性中μxUy(n) = j*P(i,j,n)+Uy(n); %相关性中μyendend
end
for n = 1:mmfor i = 1:kkfor j = 1:lldeltaX(n) = (i-Ux(n))^2*P(i,j,n)+deltaX(n); %相关性中σxdeltaY(n) = (j-Uy(n))^2*P(i,j,n)+deltaY(n); %相关性中σyC(n) = i*j*P(i,j,n)+C(n);endendC(n) = (C(n)-Ux(n)*Uy(n))/deltaX(n)/deltaY(n); %相关性
end%--------------------------------------------------------------------------
%求能量、熵、惯性矩、相关的均值和标准差作为最终8维纹理特征
%--------------------------------------------------------------------------
a1 = mean(E)
b1 = sqrt(cov(E))a2 = mean(H)
b2 = sqrt(cov(H))a3 = mean(I)
b3 = sqrt(cov(I))a4 = mean(C)
b4 = sqrt(cov(C))sprintf('0,45,90,135方向上的能量依次为： %f, %f, %f, %f',E(1),E(2),E(3),E(4))  % 输出数据;
sprintf('0,45,90,135方向上的熵依次为： %f, %f, %f, %f',H(1),H(2),H(3),H(4))  % 输出数据;
sprintf('0,45,90,135方向上的惯性矩依次为： %f, %f, %f, %f',I(1),I(2),I(3),I(4))  % 输出数据;
sprintf('0,45,90,135方向上的相关性依次为： %f, %f, %f, %f',C(1),C(2),C(3),C(4))  % 输出数据;

下面给出的是默认的设置下求四个方向的灰度共生矩阵，NumLevels=8.

gray = [1 1 5 6 8  2 3 5 7 1  4 5 7 1 2  8 5 1 2 5];
offsets = [0 1;-1 1;-1 0;-1 -1];
[GLCMS,SI] = graycomatrix(gray,'GrayLimits',[],'Of',offsets)GLCMS(:,:,1) =1     2     0     0     1     0     0     00     0     1     0     1     0     0     00     0     0     0     1     0     0     00     0     0     0     1     0     0     01     0     0     0     0     1     2     00     0     0     0     0     0     0     12     0     0     0     0     0     0     00     0     0     0     1     0     0     0GLCMS(:,:,2) =2     0     0     0     0     0     0     01     1     0     0     0     0     0     00     0     0     0     1     0     0     00     0     1     0     0     0     0     00     0     0     0     1     1     1     00     0     0     0     0     0     0     00     0     0     0     0     0     1     10     0     0     0     1     0     0     0GLCMS(:,:,3) =0     0     0     0     0     0     2     13     0     0     0     0     0     0     01     0     0     0     0     0     0     00     1     0     0     0     0     0     00     1     1     0     2     0     0     00     0     0     0     0     0     0     00     0     0     0     1     1     0     00     0     0     1     0     0     0     0GLCMS(:,:,4) =0     0     0     0     2     1     0     00     0     0     0     0     0     2     01     0     0     0     0     0     0     00     0     0     0     0     0     0     02     1     0     1     0     0     0     00     0     0     0     0     0     0     00     0     1     0     1     0     0     00     0     0     0     0     0     0     0SI =1     1     5     6     82     3     5     7     14     5     7     1     28     5     1     2     5

下面给出更多的关于灰度共生矩阵的特征

I = imread('circuit.tif');
GLCM2 = graycomatrix(I,'GrayLimits',[],'Offset',[0 1;-1 1;-1 0;-1 -1]);
stats = GLCM_Features1(GLCM2,0)
I = imread('circuit.tif');
AllGLCMFeatureb= caluateglcmfeature(I);
function [newglcm] = caluateglcmfeature(I);
GLCM2 = graycomatrix(I,'GrayLimits',[],'Offset',[0 1;-1 1;-1 0;-1 -1]);
stats = GLCM_Features1(GLCM2,0)
newstats = struct2cell(stats);
[statsx,statsy] = size(newstats);
newglcm = [];
for i = 1:statsxelement = [];newelement = [];glcm = [];element = newstats(i,1);newelement = element{1,1};average = mean(newelement);variance = sqrt(cov(newelement));glcm = [newelement average variance];newglcm = [newglcm glcm];
end
end
% http://www.mathworks.com/matlabcentral/fileexchange/22187-glcm-texture-features
% This code from the upper website but we have changed some.
function [out] = GLCM_Features1(glcmin,pairs)
% GLCM_Features1 helps to calculate the features from the different GLCMs
% that are input to the function. The GLCMs are stored in a i x j x n
% matrix, where n is the number of GLCMs calculated usually due to the
% different orientation and displacements used in the algorithm. Usually
% the values i and j are equal to 'NumLevels' parameter of the GLCM
% computing function graycomatrix(). Note that matlab quantization values
% belong to the set {1,..., NumLevels} and not from {0,...,(NumLevels-1)}
% as provided in some references
% http://www.mathworks.com/access/helpdesk/help/toolbox/images/graycomatrix
% .html
% 
% Although there is a function graycoprops() in Matlab Image Processing
% Toolbox that computes four parameters Contrast, Correlation, Energy,
% and Homogeneity. The paper by Haralick suggests a few more parameters
% that are also computed here. The code is not fully vectorized and hence
% is not an efficient implementation but it is easy to add new features
% based on the GLCM using this code. Takes care of 3 dimensional glcms
% (multiple glcms in a single 3D array)
% 
% If you find that the values obtained are different from what you expect 
% or if you think there is a different formula that needs to be used 
% from the ones used in this code please let me know. 
% A few questions which I have are listed in the link 
% http://www.mathworks.com/matlabcentral/newsreader/view_thread/239608
%
% I plan to submit a vectorized version of the code later and provide 
% updates based on replies to the above link and this initial code. 
%
% Features computed 
% Autocorrelation: [2]                      (out.autoc)
% Contrast: matlab/[1,2]                    (out.contr)
% Correlation: matlab                       (out.corrm)
% Correlation: [1,2]                        (out.corrp)
% Cluster Prominence: [2]                   (out.cprom)
% Cluster Shade: [2]                        (out.cshad)
% Dissimilarity: [2]                        (out.dissi)
% Energy: matlab / [1,2]                    (out.energ)
% Entropy: [2]                              (out.entro)
% Homogeneity: matlab                       (out.homom)
% Homogeneity: [2]                          (out.homop)
% Maximum probability: [2]                  (out.maxpr)
% Sum of sqaures: Variance [1]              (out.sosvh)
% Sum average [1]                           (out.savgh)
% Sum variance [1]                          (out.svarh)
% Sum entropy [1]                           (out.senth)
% Difference variance [1]                   (out.dvarh)
% Difference entropy [1]                    (out.denth)
% Information measure of correlation1 [1]   (out.inf1h)
% Informaiton measure of correlation2 [1]   (out.inf2h)
% Inverse difference (INV) is homom [3]     (out.homom)
% Inverse difference normalized (INN) [3]   (out.indnc) 
% Inverse difference moment normalized [3]  (out.idmnc)
%
% The maximal correlation coefficient was not calculated due to
% computational instability 
% http://murphylab.web.cmu.edu/publications/boland/boland_node26.html
%
% Formulae from MATLAB site (some look different from
% the paper by Haralick but are equivalent and give same results)
% Example formulae: 
% Contrast = sum_i(sum_j(  (i-j)^2 * p(i,j) ) ) (same in matlab/paper)
% Correlation = sum_i( sum_j( (i - u_i)(j - u_j)p(i,j)/(s_i.s_j) ) ) (m)
% Correlation = sum_i( sum_j( ((ij)p(i,j) - u_x.u_y) / (s_x.s_y) ) ) (p[2])
% Energy = sum_i( sum_j( p(i,j)^2 ) )           (same in matlab/paper)
% Homogeneity = sum_i( sum_j( p(i,j) / (1 + |i-j|) ) ) (as in matlab)
% Homogeneity = sum_i( sum_j( p(i,j) / (1 + (i-j)^2) ) ) (as in paper)
% 
% Where:
% u_i = u_x = sum_i( sum_j( i.p(i,j) ) ) (in paper [2])
% u_j = u_y = sum_i( sum_j( j.p(i,j) ) ) (in paper [2])
% s_i = s_x = sum_i( sum_j( (i - u_x)^2.p(i,j) ) ) (in paper [2])
% s_j = s_y = sum_i( sum_j( (j - u_y)^2.p(i,j) ) ) (in paper [2])
%
% 
% Normalize the glcm:
% Compute the sum of all the values in each glcm in the array and divide 
% each element by it sum
%
% Haralick uses 'Symmetric' = true in computing the glcm
% There is no Symmetric flag in the Matlab version I use hence
% I add the diagonally opposite pairs to obtain the Haralick glcm
% Here it is assumed that the diagonally opposite orientations are paired
% one after the other in the matrix
% If the above assumption is true with respect to the input glcm then
% setting the flag 'pairs' to 1 will compute the final glcms that would result 
% by setting 'Symmetric' to true. If your glcm is computed using the
% Matlab version with 'Symmetric' flag you can set the flag 'pairs' to 0
%
% References:
% 1. R. M. Haralick, K. Shanmugam, and I. Dinstein, Textural Features of
% Image Classification, IEEE Transactions on Systems, Man and Cybernetics,
% vol. SMC-3, no. 6, Nov. 1973
% 2. L. Soh and C. Tsatsoulis, Texture Analysis of SAR Sea Ice Imagery
% Using Gray Level Co-Occurrence Matrices, IEEE Transactions on Geoscience
% and Remote Sensing, vol. 37, no. 2, March 1999.
% 3. D A. Clausi, An analysis of co-occurrence texture statistics as a
% function of grey level quantization, Can. J. Remote Sensing, vol. 28, no.
% 1, pp. 45-62, 2002
% 4. http://murphylab.web.cmu.edu/publications/boland/boland_node26.html
%
%
% Example:
%
% Usage is similar to graycoprops() but needs extra parameter 'pairs' apart
% from the GLCM as input
% I = imread('circuit.tif');
% GLCM2 = graycomatrix(I,'GrayLimits',[],'Offset',[0 1;-1 1;-1 0;-1 -1]);
% stats = GLCM_Features1(GLCM2,0)
% The output is a structure containing all the parameters for the different
% GLCMs
%
% [Avinash Uppuluri: avinash_uv@yahoo.com: Last modified: 11/20/08]% If 'pairs' not entered: set pairs to 0 
if ((nargin > 2) || (nargin == 0))error('Too many or too few input arguments. Enter GLCM and pairs.');
elseif ( (nargin == 2) ) if ((size(glcmin,1) <= 1) || (size(glcmin,2) <= 1))error('The GLCM should be a 2-D or 3-D matrix.');elseif ( size(glcmin,1) ~= size(glcmin,2) )error('Each GLCM should be square with NumLevels rows and NumLevels cols');end    
elseif (nargin == 1) % only GLCM is enteredpairs = 0; % default is numbers and input 1 for percentageif ((size(glcmin,1) <= 1) || (size(glcmin,2) <= 1))error('The GLCM should be a 2-D or 3-D matrix.');elseif ( size(glcmin,1) ~= size(glcmin,2) )error('Each GLCM should be square with NumLevels rows and NumLevels cols');end    
endformat long e
if (pairs == 1)newn = 1;for nglcm = 1:2:size(glcmin,3)glcm(:,:,newn)  = glcmin(:,:,nglcm) + glcmin(:,:,nglcm+1);newn = newn + 1;end
elseif (pairs == 0)glcm = glcmin;
endsize_glcm_1 = size(glcm,1);
size_glcm_2 = size(glcm,2);
size_glcm_3 = size(glcm,3);% checked 
out.autoc = zeros(1,size_glcm_3); % Autocorrelation: [2] 
out.contr = zeros(1,size_glcm_3); % Contrast: matlab/[1,2]
out.corrm = zeros(1,size_glcm_3); % Correlation: matlab
out.corrp = zeros(1,size_glcm_3); % Correlation: [1,2]
out.cprom = zeros(1,size_glcm_3); % Cluster Prominence: [2]
out.cshad = zeros(1,size_glcm_3); % Cluster Shade: [2]
out.dissi = zeros(1,size_glcm_3); % Dissimilarity: [2]
out.energ = zeros(1,size_glcm_3); % Energy: matlab / [1,2]
out.entro = zeros(1,size_glcm_3); % Entropy: [2]
out.homom = zeros(1,size_glcm_3); % Homogeneity: matlab
out.homop = zeros(1,size_glcm_3); % Homogeneity: [2]
out.maxpr = zeros(1,size_glcm_3); % Maximum probability: [2]out.sosvh = zeros(1,size_glcm_3); % Sum of sqaures: Variance [1]
out.savgh = zeros(1,size_glcm_3); % Sum average [1]
out.svarh = zeros(1,size_glcm_3); % Sum variance [1]
out.senth = zeros(1,size_glcm_3); % Sum entropy [1]
out.dvarh = zeros(1,size_glcm_3); % Difference variance [4]
%out.dvarh2 = zeros(1,size_glcm_3); % Difference variance [1]
out.denth = zeros(1,size_glcm_3); % Difference entropy [1]
out.inf1h = zeros(1,size_glcm_3); % Information measure of correlation1 [1]
out.inf2h = zeros(1,size_glcm_3); % Informaiton measure of correlation2 [1]
%out.mxcch = zeros(1,size_glcm_3);% maximal correlation coefficient [1]
%out.invdc = zeros(1,size_glcm_3);% Inverse difference (INV) is homom [3]
out.indnc = zeros(1,size_glcm_3); % Inverse difference normalized (INN) [3]
out.idmnc = zeros(1,size_glcm_3); % Inverse difference moment normalized [3]% correlation with alternate definition of u and s
%out.corrm2 = zeros(1,size_glcm_3); % Correlation: matlab
%out.corrp2 = zeros(1,size_glcm_3); % Correlation: [1,2]glcm_sum  = zeros(size_glcm_3,1);
glcm_mean = zeros(size_glcm_3,1);
glcm_var  = zeros(size_glcm_3,1);% http://www.fp.ucalgary.ca/mhallbey/glcm_mean.htm confuses the range of 
% i and j used in calculating the means and standard deviations.
% As of now I am not sure if the range of i and j should be [1:Ng] or
% [0:Ng-1]. I am working on obtaining the values of mean and std that get
% the values of correlation that are provided by matlab.
u_x = zeros(size_glcm_3,1);
u_y = zeros(size_glcm_3,1);
s_x = zeros(size_glcm_3,1);
s_y = zeros(size_glcm_3,1);% % alternate values of u and s
% u_x2 = zeros(size_glcm_3,1);
% u_y2 = zeros(size_glcm_3,1);
% s_x2 = zeros(size_glcm_3,1);
% s_y2 = zeros(size_glcm_3,1);% checked p_x p_y p_xplusy p_xminusy
p_x = zeros(size_glcm_1,size_glcm_3); % Ng x #glcms[1]  
p_y = zeros(size_glcm_2,size_glcm_3); % Ng x #glcms[1]
p_xplusy = zeros((size_glcm_1*2 - 1),size_glcm_3); %[1]
p_xminusy = zeros((size_glcm_1),size_glcm_3); %[1]
% checked hxy hxy1 hxy2 hx hy
hxy  = zeros(size_glcm_3,1);
hxy1 = zeros(size_glcm_3,1);
hx   = zeros(size_glcm_3,1);
hy   = zeros(size_glcm_3,1);
hxy2 = zeros(size_glcm_3,1);%Q    = zeros(size(glcm));for k = 1:size_glcm_3 % number glcmsglcm_sum(k) = sum(sum(glcm(:,:,k)));glcm(:,:,k) = glcm(:,:,k)./glcm_sum(k); % Normalize each glcmglcm_mean(k) = mean2(glcm(:,:,k)); % compute mean after normglcm_var(k)  = (std2(glcm(:,:,k)))^2;for i = 1:size_glcm_1for j = 1:size_glcm_2out.contr(k) = out.contr(k) + (abs(i - j))^2.*glcm(i,j,k);out.dissi(k) = out.dissi(k) + (abs(i - j)*glcm(i,j,k));out.energ(k) = out.energ(k) + (glcm(i,j,k).^2);out.entro(k) = out.entro(k) - (glcm(i,j,k)*log(glcm(i,j,k) + eps));out.homom(k) = out.homom(k) + (glcm(i,j,k)/( 1 + abs(i-j) ));out.homop(k) = out.homop(k) + (glcm(i,j,k)/( 1 + (i - j)^2));% [1] explains sum of squares variance with a mean value;% the exact definition for mean has not been provided in % the reference: I use the mean of the entire normalized glcm out.sosvh(k) = out.sosvh(k) + glcm(i,j,k)*((i - glcm_mean(k))^2);%out.invdc(k) = out.homom(k);out.indnc(k) = out.indnc(k) + (glcm(i,j,k)/( 1 + (abs(i-j)/size_glcm_1) ));out.idmnc(k) = out.idmnc(k) + (glcm(i,j,k)/( 1 + ((i - j)/size_glcm_1)^2));u_x(k)          = u_x(k) + (i)*glcm(i,j,k); % changed 10/26/08u_y(k)          = u_y(k) + (j)*glcm(i,j,k); % changed 10/26/08% code requires that Nx = Ny % the values of the grey levels range from 1 to (Ng) endendout.maxpr(k) = max(max(glcm(:,:,k)));
end
% glcms have been normalized:
% The contrast has been computed for each glcm in the 3D matrix
% (tested) gives similar results to the matlab functionfor k = 1:size_glcm_3for i = 1:size_glcm_1for j = 1:size_glcm_2p_x(i,k) = p_x(i,k) + glcm(i,j,k); p_y(i,k) = p_y(i,k) + glcm(j,i,k); % taking i for j and j for iif (ismember((i + j),[2:2*size_glcm_1])) p_xplusy((i+j)-1,k) = p_xplusy((i+j)-1,k) + glcm(i,j,k);endif (ismember(abs(i-j),[0:(size_glcm_1-1)])) p_xminusy((abs(i-j))+1,k) = p_xminusy((abs(i-j))+1,k) +...glcm(i,j,k);endendend%     % consider u_x and u_y and s_x and s_y as means and standard deviations
%     % of p_x and p_y
%     u_x2(k) = mean(p_x(:,k));
%     u_y2(k) = mean(p_y(:,k));
%     s_x2(k) = std(p_x(:,k));
%     s_y2(k) = std(p_y(:,k));end% marginal probabilities are now available [1]
% p_xminusy has +1 in index for matlab (no 0 index)
% computing sum average, sum variance and sum entropy:
for k = 1:(size_glcm_3)for i = 1:(2*(size_glcm_1)-1)out.savgh(k) = out.savgh(k) + (i+1)*p_xplusy(i,k);% the summation for savgh is for i from 2 to 2*Ng hence (i+1)out.senth(k) = out.senth(k) - (p_xplusy(i,k)*log(p_xplusy(i,k) + eps));endend
% compute sum variance with the help of sum entropy
for k = 1:(size_glcm_3)for i = 1:(2*(size_glcm_1)-1)out.svarh(k) = out.svarh(k) + (((i+1) - out.senth(k))^2)*p_xplusy(i,k);% the summation for savgh is for i from 2 to 2*Ng hence (i+1)endend
% compute difference variance, difference entropy, 
for k = 1:size_glcm_3
% out.dvarh2(k) = var(p_xminusy(:,k));
% but using the formula in 
% http://murphylab.web.cmu.edu/publications/boland/boland_node26.html
% we have for dvarhfor i = 0:(size_glcm_1-1)out.denth(k) = out.denth(k) - (p_xminusy(i+1,k)*log(p_xminusy(i+1,k) + eps));out.dvarh(k) = out.dvarh(k) + (i^2)*p_xminusy(i+1,k);end
end% compute information measure of correlation(1,2) [1]
for k = 1:size_glcm_3hxy(k) = out.entro(k);for i = 1:size_glcm_1for j = 1:size_glcm_2hxy1(k) = hxy1(k) - (glcm(i,j,k)*log(p_x(i,k)*p_y(j,k) + eps));hxy2(k) = hxy2(k) - (p_x(i,k)*p_y(j,k)*log(p_x(i,k)*p_y(j,k) + eps));
%             for Qind = 1:(size_glcm_1)
%                 Q(i,j,k) = Q(i,j,k) +...
%                     ( glcm(i,Qind,k)*glcm(j,Qind,k) / (p_x(i,k)*p_y(Qind,k)) ); 
%             endendhx(k) = hx(k) - (p_x(i,k)*log(p_x(i,k) + eps));hy(k) = hy(k) - (p_y(i,k)*log(p_y(i,k) + eps));endout.inf1h(k) = ( hxy(k) - hxy1(k) ) / ( max([hx(k),hy(k)]) );out.inf2h(k) = ( 1 - exp( -2*( hxy2(k) - hxy(k) ) ) )^0.5;
%     eig_Q(k,:)   = eig(Q(:,:,k));
%     sort_eig(k,:)= sort(eig_Q(k,:),'descend');
%     out.mxcch(k) = sort_eig(k,2)^0.5;
% The maximal correlation coefficient was not calculated due to
% computational instability 
% http://murphylab.web.cmu.edu/publications/boland/boland_node26.html
endcorm = zeros(size_glcm_3,1);
corp = zeros(size_glcm_3,1);
% using http://www.fp.ucalgary.ca/mhallbey/glcm_variance.htm for s_x s_y
for k = 1:size_glcm_3for i = 1:size_glcm_1for j = 1:size_glcm_2s_x(k)  = s_x(k)  + (((i) - u_x(k))^2)*glcm(i,j,k);s_y(k)  = s_y(k)  + (((j) - u_y(k))^2)*glcm(i,j,k);corp(k) = corp(k) + ((i)*(j)*glcm(i,j,k));corm(k) = corm(k) + (((i) - u_x(k))*((j) - u_y(k))*glcm(i,j,k));out.cprom(k) = out.cprom(k) + (((i + j - u_x(k) - u_y(k))^4)*...glcm(i,j,k));out.cshad(k) = out.cshad(k) + (((i + j - u_x(k) - u_y(k))^3)*...glcm(i,j,k));endend% using http://www.fp.ucalgary.ca/mhallbey/glcm_variance.htm for s_x% s_y : This solves the difference in value of correlation and might be% the right value of standard deviations required % According to this website there is a typo in [2] which provides% values of variance instead of the standard deviation hence a square% root is required as done below:s_x(k) = s_x(k) ^ 0.5;s_y(k) = s_y(k) ^ 0.5;out.autoc(k) = corp(k);out.corrp(k) = (corp(k) - u_x(k)*u_y(k))/(s_x(k)*s_y(k));out.corrm(k) = corm(k) / (s_x(k)*s_y(k));
%     % alternate values of u and s
%     out.corrp2(k) = (corp(k) - u_x2(k)*u_y2(k))/(s_x2(k)*s_y2(k));
%     out.corrm2(k) = corm(k) / (s_x2(k)*s_y2(k));
end
%--------------------------------------------------------------------------% Here the formula in the paper out.corrp and the formula in matlab
% out.corrm are equivalent as confirmed by the similar results obtained% % The papers have a slightly different formular for Contrast
% % I have tested here to find this formula in the papers provides the 
% % same results as the formula provided by the matlab function for 
% % Contrast (Hence this part has been commented)
% out.contrp = zeros(size_glcm_3,1);
% contp = 0;
% Ng = size_glcm_1;
% for k = 1:size_glcm_3
%     for n = 0:(Ng-1)
%         for i = 1:Ng
%             for j = 1:Ng
%                 if (abs(i-j) == n)
%                     contp = contp + glcm(i,j,k);
%                 end
%             end
%         end
%         out.contrp(k) = out.contrp(k) + n^2*contp;
%         contp = 0;
%     end
%     
% end%       GLCM Features (Soh, 1999; Haralick, 1973; Clausi 2002)
%           f1. Uniformity / Energy / Angular Second Moment (done)
%           f2. Entropy (done)
%           f3. Dissimilarity (done)
%           f4. Contrast / Inertia (done)
%           f5. Inverse difference    
%           f6. correlation
%           f7. Homogeneity / Inverse difference moment
%           f8. Autocorrelation
%           f9. Cluster Shade
%          f10. Cluster Prominence
%          f11. Maximum probability
%          f12. Sum of Squares
%          f13. Sum Average
%          f14. Sum Variance
%          f15. Sum Entropy
%          f16. Difference variance
%          f17. Difference entropy
%          f18. Information measures of correlation (1)
%          f19. Information measures of correlation (2)
%          f20. Maximal correlation coefficient
%          f21. Inverse difference normalized (INN)
%          f22. Inverse difference moment normalized (IDN)

本文来自互联网用户投稿，该文观点仅代表作者本人，不代表本站立场。本站仅提供信息存储空间服务，不拥有所有权，不承担相关法律责任。如若转载，请注明出处：http://www.mzph.cn/news/246996.shtml

如若内容造成侵权/违法违规/事实不符，请联系多彩编程网进行投诉反馈email:809451989@qq.com，一经查实，立即删除！

相关文章

机器学习之 weka学习（一）weka介绍，安装和配置环境变量

机器学习之 weka学习（一）weka介绍，安装和配置环境变量

本部分详情可查看博客http://blog.csdn.net/u011067360/article/details/20844443 数据挖掘开源软件&#xff1a;WEKA基础教程 Weka简介&#xff1a; Weka是由新西兰怀卡托大学开发的智能分析系统&#xff08;Waikato Environment for Knowledge Analysis&#xff09; 。在怀…
阅读更多...
第一章：线性空间和线性变换

第一章：线性空间和线性变换

转载于:https://www.cnblogs.com/invisible2/p/11514817.html
阅读更多...
国外十大高校人工智能实验室及其代表性人物一览

国外十大高校人工智能实验室及其代表性人物一览

本文转自&#xff1a; http://toutiao.com/a6293031494186107137/?tt_frommobile_qq&utm_campaignclient_share&appnews_article&utm_sourcemobile_qq&iid4325464459&utm_mediumtoutiao_android 高校建立的实验室与大公司有所不同&#xff0c;其研究项目…
阅读更多...
java 基础安装和Tomcat8配置

java 基础安装和Tomcat8配置

初识 java&#xff0c;基础安装的说明。 下载 在oracle官网一般在同一个java版本会提供2个版本&#xff0c; 一个是Java SE Development Kit 7u80&#xff0c;此版本包含JDK开发环境版本&#xff1b; 另外一个是 Java SE Runtime Environment 7u80&#xff0c;此为只包含JR…
阅读更多...
matlab内存溢出的解决方案

matlab内存溢出的解决方案

&#xff08;1&#xff09; 增加虚拟内存&#xff1a;cmd -> taskmgr 打开任务管理器&#xff0c;查看物理内存和虚拟内存&#xff0c;可观察matlab在运行过程中是否超过物理内存和虚拟内存。若超过&#xff0c;增加虚拟内存的方法是不可行的。物理内存不足的时候可以通过将…
阅读更多...
c++MMMMM:oo

c++MMMMM:oo

1.union&#xff0c;struct和class的区别 转载于:https://www.cnblogs.com/invisible2/p/11524465.html
阅读更多...
matlab调用Java程序时出现 Java.lang.OutOfMemoryErrot: GC overhead limit exceeded

matlab调用Java程序时出现 Java.lang.OutOfMemoryErrot: GC overhead limit exceeded

matlab调用Java程序时出现 java.lang.OutOfMemoryError: GC overhead limit exceeded JDK1.6.0_37和JDK_1.7.0_60版本&#xff0c;这2个版本中JVM默认启动的时候-XX:UseGCOverheadLimit&#xff0c;即启用了该特性。这其实是JVM的一种推断&#xff0c;如果垃圾回收耗费了98%的…
阅读更多...
第94：受限玻尔兹曼机

第94：受限玻尔兹曼机

转载于:https://www.cnblogs.com/invisible2/p/11565179.html
阅读更多...
安装完Ubuntu桌面后要做的(待续)

安装完Ubuntu桌面后要做的(待续)

1. 为了快速而顺畅的更新&#xff0c;打开终端并输入以下命令来让系统使用新软件库&#xff1a; $ sudo apt-get update 2. 更改系统外观和行为 如果你想要更改桌面背景或图标大小&#xff0c;依次打开System Settings –> Appearance –> Look&#xff0c;并对桌面进…
阅读更多...
算法第二章上机实践报告

算法第二章上机实践报告

一、实践题目 改写二分搜索算法 二、问题描述 这道题目主要是考验同学们在熟练掌握二分搜索法的前提下&#xff0c;对二分搜索的结构和运用有一个更加深刻的掌握。首先是要了解二分搜索的结构&#xff0c;其次&#xff0c;要了解二分搜索中的分治方法每一个步骤的用意&#xff…
阅读更多...
windows远程登录 ubuntu Linux 系统及互连共享桌面

windows远程登录 ubuntu Linux 系统及互连共享桌面

预备工作 #开启防火墙端口 sudo ufw allow 3389#安装ssh sudo apt-get install openssh-server一、windows直连Ubuntu16.04共享桌面 1、打开终端&#xff0c;安装xrdp,vncserver sudo apt-get install xrdp vnc4server xbase-clients2、安装desktop sharing&#xff08;Ubuntu…
阅读更多...
RAID详解

RAID详解

一、raid什么意思&#xff1f; RAID是“Redundant Array of Independent Disk”的缩写&#xff0c;中文翻译过来通俗的讲就是磁盘阵列的意思&#xff0c;也就是说RAID就是把硬盘做成一个阵列&#xff0c;而阵列也就是把硬盘进行组合配置起来&#xff0c;做为一个整体进行管理&a…
阅读更多...
装windows和Linux系统时找不到硬盘，pe安装系统没有出现磁盘，不能识别磁盘

装windows和Linux系统时找不到硬盘，pe安装系统没有出现磁盘，不能识别磁盘

装win7的时候&#xff0c;我们使用U盘装系统&#xff0c;找不到硬盘&#xff0c; 或者使用光盘装系统时 会出现 缺少所需的CD/DVD驱动器设备驱动程序 然后找遍整个硬盘/光盘也找不到合适的驱动&#xff0c;安装无法继续。 解决方法&#xff1a; ACHI模式下&#xff0c;PE里…
阅读更多...
Linux 服务器上建立用户并分配权限

Linux 服务器上建立用户并分配权限

查看用户 whoami #要查看当前登录用户的用户名 who am i #表示打开当前伪终端的用户的用户名 who mom likes who 命令其它常用参数 参数 说明 -a 打印能打印的全部 -d 打印死掉的进程 -m 同am i,mom likes -q 打印当前登录用户数及用户名 -u 打印当前登录用户登录信…
阅读更多...
LSTM

LSTM

具体推导公式为&#xff1a; https://zybuluo.com/hanbingtao/note/581764 转载于:https://www.cnblogs.com/invisible2/p/11593270.html
阅读更多...
查看windows显卡内存详细信息

查看windows显卡内存详细信息

方式一&#xff1a; 打开运行&#xff08;WindowsR&#xff09;&#xff0c;输入DXDIAG&#xff0c;打开DirectX诊断工具 可以看到显卡是AMD Radeon HD 7000 series&#xff0c;但是没有具体显卡信息&#xff0c;这个型号是AMD在2013年主推的ATI的入门级独显系列&#xff0c;其…
阅读更多...
从服务器上传和下载文件方法

从服务器上传和下载文件方法

1. ssh 安装SSH Secure Shell Client客户端 下载链接 http://download.csdn.net/detail/jiandanjinxin/9755684 使用方法参考主页 http://www.cnblogs.com/wxjnew/archive/2013/06/05/3118808.html http://www.cnblogs.com/pingzhanga/p/5126885.html 导致此问题的原因是…
阅读更多...
Deep Learning运行所需的硬件配置（转）

Deep Learning运行所需的硬件配置（转）

A Full Hardware Guide to Deep Learning 2015-03-09 by Tim Dettmers 304 Comments 转自&#xff1a;http://timdettmers.com/2015/03/09/deep-learning-hardware-guide/ Deep Learning is very computationally intensive, so you will need a fast CPU with many cores, ri…
阅读更多...
DBUtils

DBUtils

概述 DBUtils是Java编程中的数据库操作实用工具&#xff0c;小巧简单实用。 DBUtils封装了对JDBC的操作&#xff0c;简化了JDBC操作&#xff0c;可以少写代码。 DBUtils三个核心功能介绍 QueryRunner中提供对sql语句操作的APIResultSetHandler接口&#xff0c;用于定义select操…
阅读更多...
windows7 下vmware workstation 12安装Ubuntu16.04虚拟机及安装和共享文件夹

windows7 下vmware workstation 12安装Ubuntu16.04虚拟机及安装和共享文件夹

关于安装虚拟机&#xff0c;具体细节可参考下文&#xff08;已测试可执行&#xff09; vmware workstation 12 安装 ubuntu kylin 16.04虚拟机 下面就给出在虚拟机Ubuntu16.04下安装VMware Tools&#xff0c;是为了实现目标主机和虚拟机之间的通讯。 1.打开虚拟机之后&#…
阅读更多...
推荐文章
最新文章

Copyright @ 2022~2023