113. 路径总和 II

文章目录

• • • [113. 路径总和 II](https://leetcode.cn/problems/path-sum-ii/)
    • • 一、题目
      • 二、题解
      • • 方法一：递归
        • 另一种递归版本
        • 方法二：迭代

---

一、题目

给你二叉树的根节点 root 和一个整数目标和 targetSum ，找出所有 从根节点到叶子节点 路径总和等于给定目标和的路径。

叶子节点 是指没有子节点的节点。

示例 1：

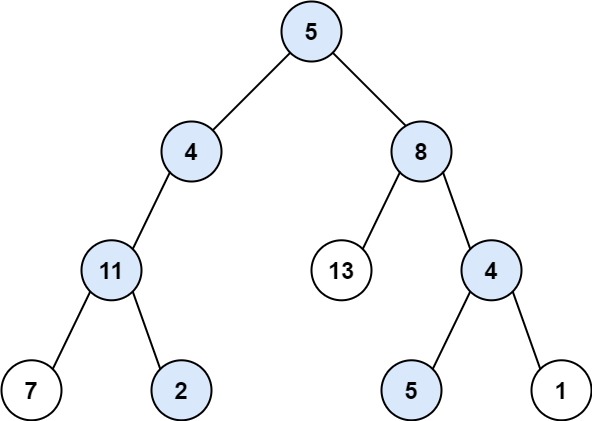
输入：root = [5,4,8,11,null,13,4,7,2,null,null,5,1], targetSum = 22
输出：[[5,4,11,2],[5,8,4,5]]

示例 2：

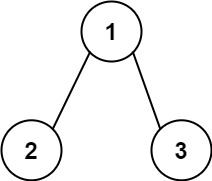
输入：root = [1,2,3], targetSum = 5
输出：[]

示例 3：

输入：root = [1,2], targetSum = 0
输出：[]

提示：

• 树中节点总数在范围 [0, 5000] 内
• -1000 <= Node.val <= 1000
• -1000 <= targetSum <= 1000

二、题解

方法一：递归

算法思路

1. 我们需要遍历二叉树中所有从根节点到叶子节点的路径，以找出满足路径和等于目标和的路径。这提示我们可以使用深度优先搜索（DFS）来遍历树的所有可能路径。

2. 我们可以在递归过程中维护一个当前路径的和 count，从根节点开始，每到一个节点，我们将该节点的值在count 上进行减法处理。

3. 如果当前节点是叶子节点（即没有左右子节点），我们检查 count 是否等于0。如果是，我们将当前路径添加到结果中。

4. 在递归过程中，我们需要传递当前路径 path，结果数组 result，当前路径的和 count，以及当前节点 root。

具体实现

class Solution {
public:void findPath(TreeNode *root, vector<vector<int>>&result, vector<int>& path, int count){if(root == nullptr) return;path.push_back(root->val);count -= root->val;if(count == 0 && !root->left && !root->right){result.push_back(path);}if(root->left){findPath(root->left, result, path, count);}if(root->right){findPath(root->right, result, path, count);}path.pop_back(); // 回溯，移除当前节点，尝试其他路径}vector<vector<int>> pathSum(TreeNode* root, int targetSum) {vector<vector<int>> result;vector<int> path;if(root == nullptr) return result;findPath(root, result, path, targetSum);return result;}
};

算法分析

• 时间复杂度：遍历整个二叉树，每个节点只访问一次，所以时间复杂度为 O(N)，其中 N 是树中的节点数。

• 空间复杂度：在递归过程中，我们使用了 path 数组来存储当前路径，最坏情况下，路径长度达到树的深度，所以空间复杂度为 O(H)，其中 H 是树的高度。在结果数组中，我们存储了满足条件的路径，最坏情况下可能会有 O(N) 个路径，所以结果数组的空间复杂度也是 O(N)。

另一种递归版本

因为cpp的特性，通过去掉 vector<int>& 中的引用符号，我可以在每个递归层次中都创建了一个新的 path 向量，这样可以确保在不同的递归路径之间不会共享相同的 path 对象，相当于进行了回溯。

/*** Definition for a binary tree node.* struct TreeNode {*     int val;*     TreeNode *left;*     TreeNode *right;*     TreeNode() : val(0), left(nullptr), right(nullptr) {}*     TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}*     TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}* };*/
class Solution {
public:void findPath(TreeNode *root, vector<vector<int>>&result, vector<int> path, int count){if(root == nullptr) return;path.push_back(root->val);count -= root->val;if(count == 0 && !root->left && !root->right){result.push_back(path);}if(root->left){findPath(root->left, result, path, count);}if(root->right){findPath(root->right, result, path, count);}}vector<vector<int>> pathSum(TreeNode* root, int targetSum) {vector<vector<int>> result;vector<int> path;if(root == nullptr) return result;int count = targetSum;findPath(root, result, path, count);return result;}
};

方法二：迭代

算法思路

1. 我们使用中序遍历来遍历树的节点，同时维护两个栈：St 用于保存遍历节点的信息，pathSt 用于保存当前路径的节点值。
2. 初始时，我们将根节点 root 和对应的路径值 root->val 入栈，表示从根节点开始的路径。
3. 在每一次循环中，我们从 St 栈中取出一个节点，同时从 pathSt 中取出与该节点对应的路径。
4. 我们检查该节点是否为叶子节点，如果是叶子节点并且路径值等于 targetSum，则将该路径保存到 result 中。
5. 如果不是叶子节点，我们将其左子节点和右子节点（如果存在的话）入栈，并更新路径值。
6. 重复以上步骤直至 St 栈为空。

具体实现

class Solution {
public:vector<vector<int>> pathSum(TreeNode* root, int targetSum) {vector<vector<int>> result; // 用于存储符合条件的路径stack<pair<TreeNode*, int>> St; // 用于深度优先搜索的栈，同时保存节点和路径和stack<vector<int>> pathSt; // 用于保存路径的栈if (root == nullptr) return result; // 处理根节点为空的情况// 初始化，将根节点和路径和入栈St.push(pair<TreeNode*, int>(root, root->val));vector<int> temp;temp.push_back(root->val);pathSt.push(temp);while (!St.empty()) {pair<TreeNode*, int> node = St.top(); St.pop(); // 弹出栈顶节点vector<int> pathT = pathSt.top(); pathSt.pop(); // 弹出栈顶路径// 如果是叶子节点且路径和等于targetSum，将路径保存到result中if (!node.first->left && !node.first->right && node.second == targetSum) {result.push_back(pathT);}// 将右子节点入栈，更新路径和，并将路径入栈if (node.first->right) {St.push(pair<TreeNode*, int>(node.first->right, node.second + node.first->right->val));vector<int> newPathT = pathT;newPathT.push_back(node.first->right->val);pathSt.push(newPathT);}// 将左子节点入栈，更新路径和，并将路径入栈if (node.first->left) {St.push(pair<TreeNode*, int>(node.first->left, node.second + node.first->left->val));vector<int> newPathT = pathT;newPathT.push_back(node.first->left->val);pathSt.push(newPathT);}}return result; // 返回所有满足条件的路径}
};

算法分析

• 时间复杂度：每个节点最多访问一次，所以时间复杂度为 O(N)，其中 N 是树的节点数。
• 空间复杂度：栈的最大空间取决于树的高度，所以空间复杂度为 O(H)，其中 H 是树的高度。

简化版本

class Solution {
public:vector<vector<int>> pathSum(TreeNode* root, int targetSum) {vector<vector<int>> result;vector<int> path;stack<TreeNode*> TreeSt;stack<vector<int>> pathSt;if(root == nullptr) return result;// 初始化TreeSt.push(root);vector<int> temp;temp.push_back(root->val);pathSt.push(temp);while(!TreeSt.empty()){TreeNode *node = TreeSt.top();TreeSt.pop();vector<int> pathT = pathSt.top();pathSt.pop();if(!node->left && !node->right && accumulate(pathT.begin(), pathT.end(), 0) == targetSum) {	//这里直接计算路径上的总和result.push_back(pathT);}if(node->right){TreeSt.push(node->right);vector<int> newPathT = pathT; newPathT.push_back(node->right->val);pathSt.push(newPathT);}if(node->left){TreeSt.push(node->left);vector<int> newPathT = pathT; newPathT.push_back(node->left->val);pathSt.push(newPathT);}}return result;}
};