《博主简介》
小伙伴们好,我是阿旭。专注于人工智能AI、python、计算机视觉相关分享研究。
✌更多学习资源,可关注公-仲-hao:【阿旭算法与机器学习】,共同学习交流~
👍感谢小伙伴们点赞、关注!
分发饼干
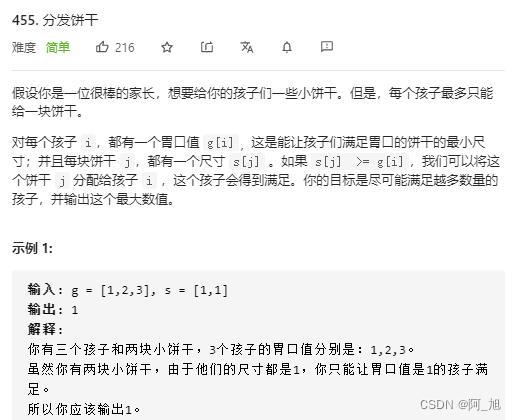
| class Solution: def findContentChildren(self, g: List[int], s: List[int]) -> int: # 贪心算法 res = 0 g.sort() s.sort() i = 0 j = 0 while i < len(g) and j < len(s): # 饼干满足胃口 if g[i] <= s[j]: res += 1 i += 1 j += 1 else: # 饼干不满足胃口,查找下一个饼干 j += 1 return res |
跳跃游戏

| class Solution: def canJump(self, nums: List[int]) -> bool: # 贪心算法 reach_index = len(nums) - 1 # 表示能够到达的索引位置 for i in range(len(nums)-1,-1,-1): # 从后往前遍历,如果满足下述条件说明能够达到当前索引 if i + nums[i] >= reach_index: reach_index = i return reach_index == 0 class Solution: def canJump(self, nums: List[int]) -> bool: if nums == [0]: return True maxDist = 0 # 能够达到的最远距离 end_index = len(nums)-1 for i, jump in enumerate(nums): # maxDist >= i表示能够达到当前索引位置,并且从当前索引开始 if maxDist >= i and i+jump > maxDist: maxDist = i+jump if maxDist >= end_index: return True return False |
跳跃游戏2

| class Solution: def jump(self, nums: List[int]) -> int: end = 0 # end 表示当前能跳的边界 maxPosition = 0 steps = 0 for i in range(len(nums) - 1): # 找能跳的最远的 maxPosition = max(maxPosition, nums[i] + i); if i == end: #遇到边界,就更新边界,并且步数加一 end = maxPosition; steps += 1 return steps; |
模拟行走机器人

| class Solution: def robotSim(self, commands: List[int], obstacles: List[List[int]]) -> int: if not commands: return 0 # 索引0,1,2,3分别表示北,东,南,西 direx = [0, 1, 0, -1] direy = [1, 0, -1, 0] curx, cury, curdire, ans = 0, 0, 0, 0 com_len, obs_len = len(commands), len(obstacles) obstacle_set = {(obstacles[i][0], obstacles[i][1]) for i in range(obs_len)} # 变为集合,使判断是否有障碍物更快
for i in range(com_len): if commands[i] == -1: # 向右转90度 curdire = (curdire +1) % 4 elif commands[i] == -2: # 向左转90度 curdire = (curdire + 3) %4 else: # 1 <= x <= 9: 向前移动x个单位长度 for j in range(commands[i]): # 试图走出一步,并判断是否遇到了障碍物 nx = curx + direx[curdire] ny = cury + direy[curdire] # 当前坐标不是障碍物,计算并存储的最大欧式距离的平方做比较 if (nx, ny) not in obstacle_set: curx = nx cury = ny ans = max(ans, curx*curx + cury*cury) else: # 是障碍点,被挡住了,停留,智能等待下一个指令,那可以跳出当前指令了。 break return ans |
关于本篇文章大家有任何建议或意见,欢迎在评论区留言交流!
觉得不错的小伙伴,感谢点赞、关注加收藏哦!
欢迎关注下方GZH:阿旭算法与机器学习,共同学习交流~


:多个agent模型)









)






