二叉树在线OJ

二叉树的构建及遍历

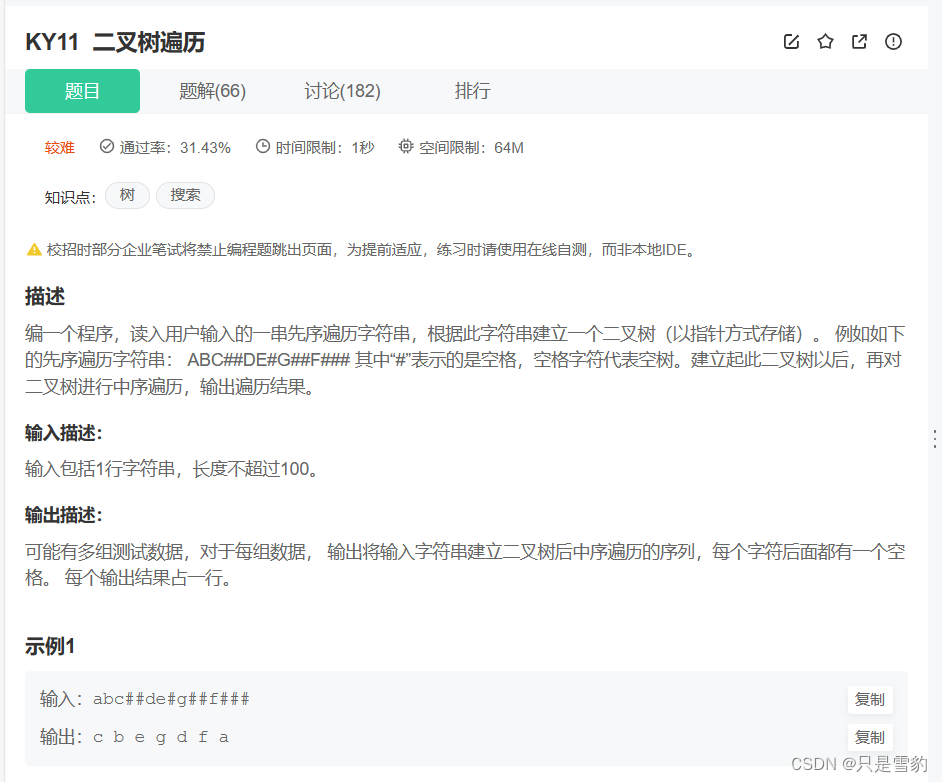
在这里插入图片描述
本题目的要求是：
输入一个数组，里面存放了若干个字符，#代表了空指针，数组中的顺序是
是先序遍历，然后要求你用中序输出
首先我们要做的就是构造结构体：

typedef struct TreeNode
{char val;struct TreeNode* left;struct TreeNode* right;
}TreeNode;

然后用先序遍历构造二叉树
当数组的首元素为#或者“\0”，即二叉树没有根节点，为空树，直接接返回
然后我们开辟新节点的空间
然后进行先序遍历构造二叉树：
首先将newnode的值置为数组首元素，同时下标count++
然后递归newnode的左子树根节点，*（count）++（count传的是地址，所以记得解引用），随后递归右子树根节点即可，最后返回newnode，就是二叉树的根节点

TreeNode* maketree(char*arr,int*count)
{if(arr[*count]=='#'||arr[*count]=='\0'){return NULL;}TreeNode* newnode = (TreeNode*)malloc(sizeof(TreeNode));newnode->val = arr[(*count)++];newnode->left = maketree(arr,count);(*count)++;newnode->right = maketree(arr,count);return newnode;
}

最后写一个中序遍历的输出：

void Inorder(TreeNode* root)
{if(root==NULL){return;}Inorder(root->left);printf("%c ",root->val);Inorder(root->right);
}

完整代码如下：

#include <stdio.h>
#include<stdlib.h>
typedef struct TreeNode
{char val;struct TreeNode* left;struct TreeNode* right;
}TreeNode;
TreeNode* maketree(char*arr,int*count)
{if(arr[*count]=='#'||arr[*count]=='\0'){return NULL;}TreeNode* newnode = (TreeNode*)malloc(sizeof(TreeNode));newnode->val = arr[(*count)++];newnode->left = maketree(arr,count);(*count)++;newnode->right = maketree(arr,count);return newnode;
}
void Inorder(TreeNode* root)
{if(root==NULL){return;}Inorder(root->left);printf("%c ",root->val);Inorder(root->right);
}int main()
{char arr[101];scanf("%s",arr);int count = 0;TreeNode* tree = maketree(arr,&count);Inorder(tree);return 0;
}

判断一颗二叉树是否是平衡二叉树

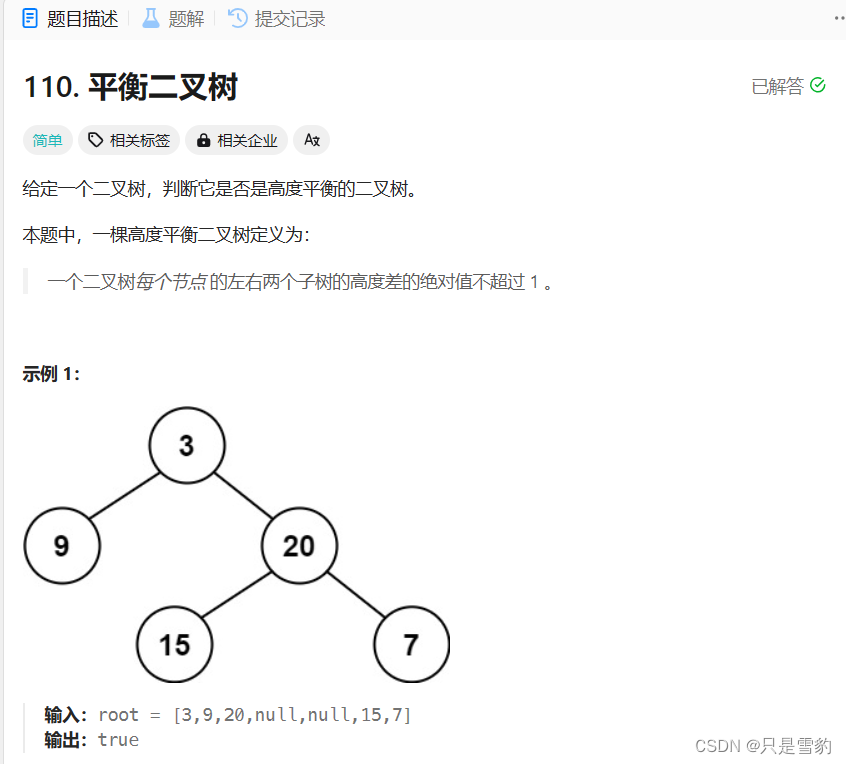
在这里插入图片描述
本题很简单了：
直接判断左子树和右子树的高度的绝对值是否小于等于1即可
同时左子树的子树和右子树的子树也要同时递归
调用求二叉树高度的函数

int height(struct TreeNode* root)
{if(root==NULL){return 0;}return fmax(height(root->left),height(root->right))+1;
}
bool isBalanced(struct TreeNode* root) 
{if(root==NULL){return true;}return fabs(height(root->left) - height(root->right)) <= 1 && isBalanced(root->left) && isBalanced(root->right);
}

另一颗树的子树

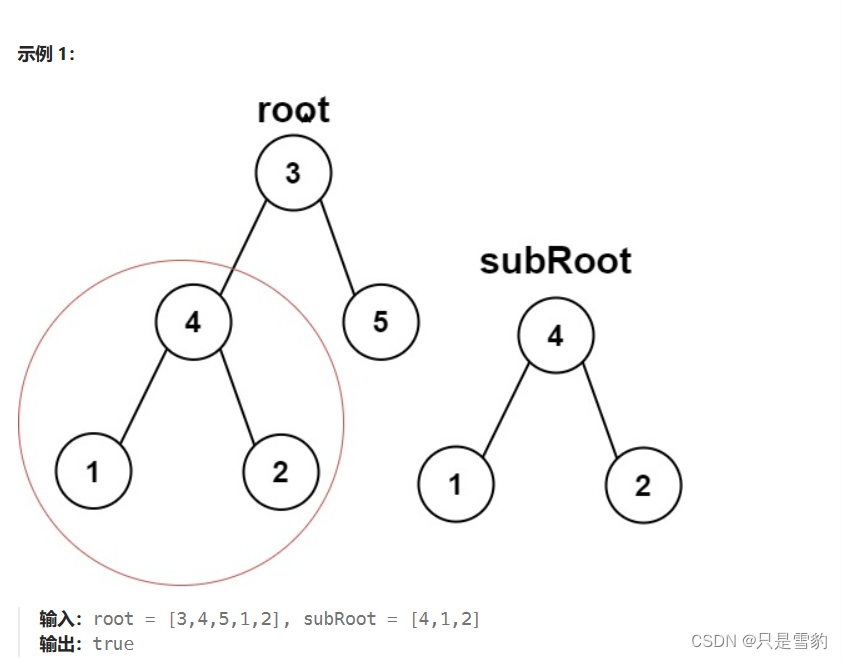
在这里插入图片描述

本题就是判断一颗树的子树是否是另一棵树
所以我们首先要做的就是构造一个判断两棵树是否相等的函数：
当两个树同时为空时也是相等的
当其中一个为空时肯定时不想同的
当root的值不等于subroot的值时肯定是不相等的

bool issame(struct TreeNode* root,struct TreeNode* subroot)
{if(root==NULL&&subroot==NULL)return true;if(root==NULL||subroot==NULL)return false;if(root->val==subroot->val){if(issame(root->left,subroot->left)&&issame(root->right,subroot->right))return true;}return false;
}

然后调用这个函数：
当root和subroot相等时也符合题意

bool isSubtree(struct TreeNode* root, struct TreeNode* subRoot)
{if(root==NULL)return false;if(issame(root,subRoot))return true;return isSubtree(root->left,subRoot)||isSubtree(root->right,subRoot);
}

对称二叉树

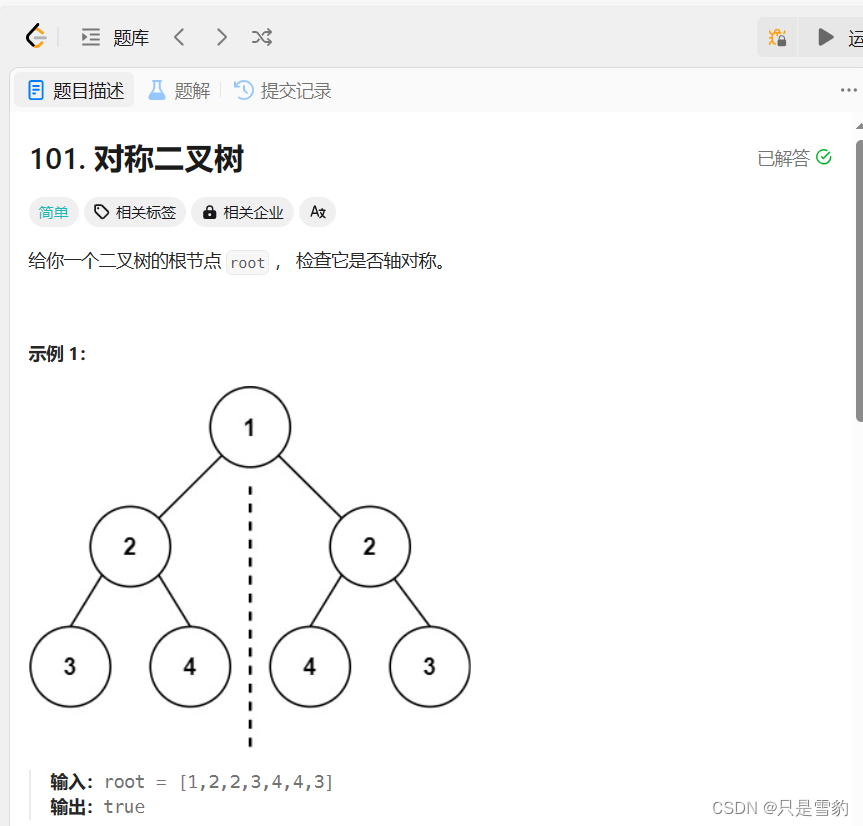
在这里插入图片描述
其实判断二叉树是否对称就是左边和右边比较，对折重合
即：
左子树和右子树相同，左子树的左子树后右子树的右子树相同
我们直接调用上一题的判断树是否相同的函数
然后拿左子树和右子树比较即可
当左子树的根节点和右子树的根节点不相等时只返回false

bool issame(struct TreeNode* rootleft,struct TreeNode* rootright)
{if(rootleft==NULL&&rootright==NULL)return true;if(rootleft==NULL||rootright==NULL)return false;if(rootleft->val==rootright->val){if(issame(rootleft->left,rootright->right)&&issame(rootleft->right,rootright->left))return true;}return false;
}
bool isSymmetric(struct TreeNode* root) 
{if(root->left==NULL&&root->right==NULL)return true;if(root->left==NULL||root->right==NULL)return false;if(root->left->val==root->right->val){if(issame(root->left,root->right))return true;}return false;
}

检查两颗树是否相同

这不简简单单，和之前的函数差不多，使用递归就是了
同时为空返回true
有一个为空返回false
两个根节点的值不相等返回false

bool isSameTree(struct TreeNode* p, struct TreeNode* q) 
{if(p==NULL&&q==NULL){return true;}if(p==NULL||q==NULL){return false;}if(p->val!=q->val){return false;}return isSameTree(p->left,q->left)&&isSameTree(p->right,q->right);
}

翻转二叉树

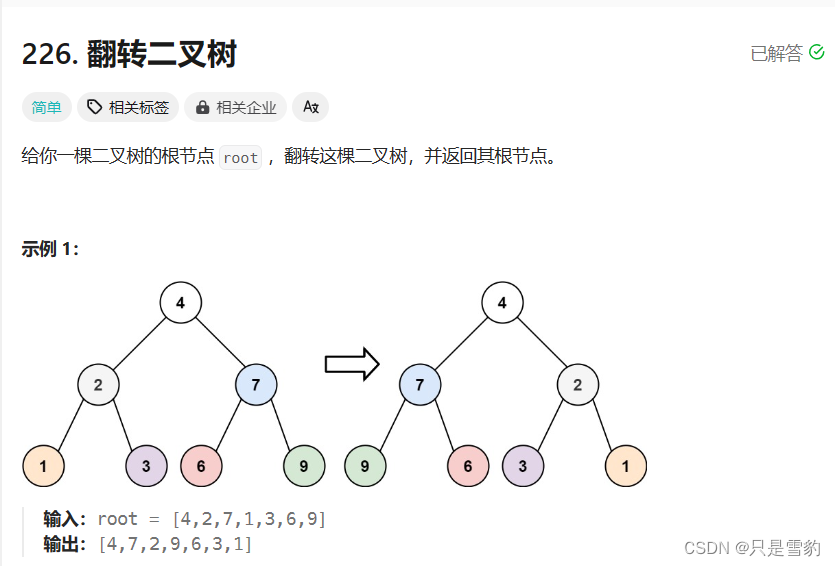
在这里插入图片描述
反转二叉树就是把左子树变成右子树，右子树变成左子树
很简单，直接先开辟空间存放，然后赋于即可

struct TreeNode* invertTree(struct TreeNode* root) 
{if (root == NULL) {return NULL;}struct TreeNode* left = invertTree(root->left);struct TreeNode* right = invertTree(root->right);root->left = right;root->right = left;return root;
}

二叉树最大深度

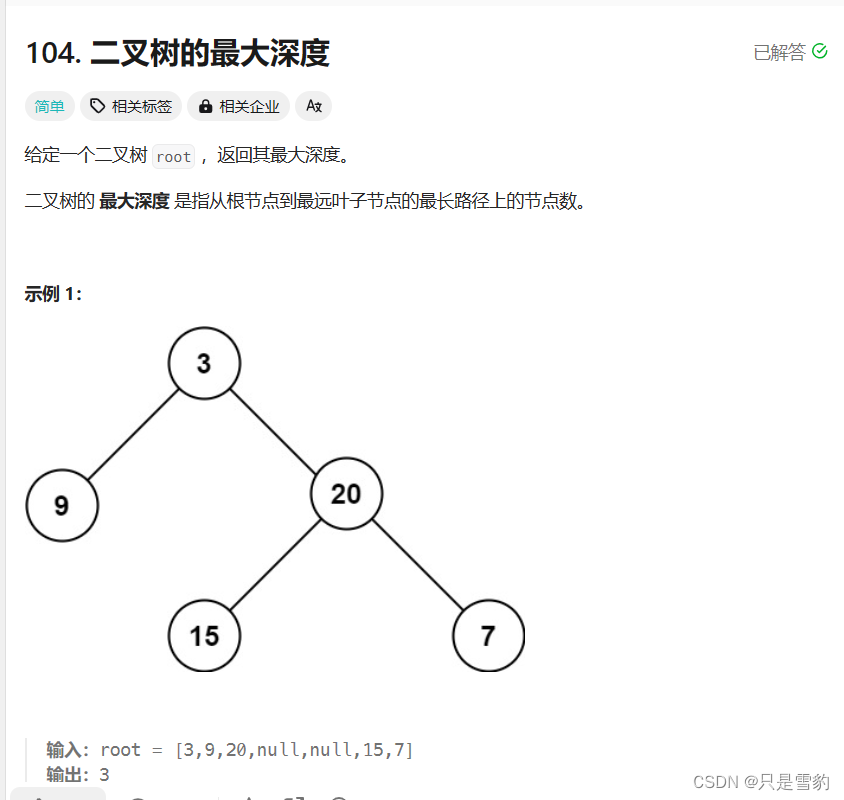
在这里插入图片描述
最大深度不就是高度吗，就是层数啊
直接递归，就是左子树和右子树中深度大的那一个+1

int maxDepth(struct TreeNode* root) 
{if(root==NULL)return 0;return fmax(maxDepth(root->left), maxDepth(root->right)) + 1;
}

单值二叉树

在这里插入图片描述
单值二叉树就是二叉树所有节点的值都相等
二叉树为空符合题意，返回true
左子树和右子树为空符合题意，只有一个节点，返回true
当有一个不为空时，不为空子树根节点和根节点不相等直接返回false
当两个子树都不为空时判断两个子树的根节点是否相等，不相等直接返回false，然后递归左子树和右子树的根节点

bool isUnivalTree(struct TreeNode* root) 
{if(root==NULL)return true;if(root->left==NULL&&root->right==NULL)return true;if(root->left==NULL||root->right==NULL){if(root->left==NULL){if(root->val!=root->right->val)return false;}if(root->right==NULL){if(root->val!=root->left->val)return false;}}if(root->left!=NULL&&root->right!=NULL){if(root->val!=root->left->val||root->val!=root->right->val)return false;}return isUnivalTree(root->left)&&isUnivalTree(root->right);
}

好了，今天的分享到这里就结束了，谢谢大家！

本文来自互联网用户投稿，该文观点仅代表作者本人，不代表本站立场。本站仅提供信息存储空间服务，不拥有所有权，不承担相关法律责任。如若转载，请注明出处：http://www.mzph.cn/news/193439.shtml

如若内容造成侵权/违法违规/事实不符，请联系多彩编程网进行投诉反馈email:809451989@qq.com，一经查实，立即删除！