第一题: 寻找满足特定条件的 e;
第一步:

第二步:
由式1.7知,给定e,p,q,就可计算出相应的RSA不动点的数目。因此设计算法步骤如下:
- 枚举找出所有与φ(n)互素的e。
- 枚举所有满足条件的e,计算RSA不动点的数目。
- 以RSA不动点的数目为键,累加变量为值,将每次的结果添加到字典中。
- 最后输出最小值的键对应的累加值。
代码以及运行结果如下,结果为399788195976:
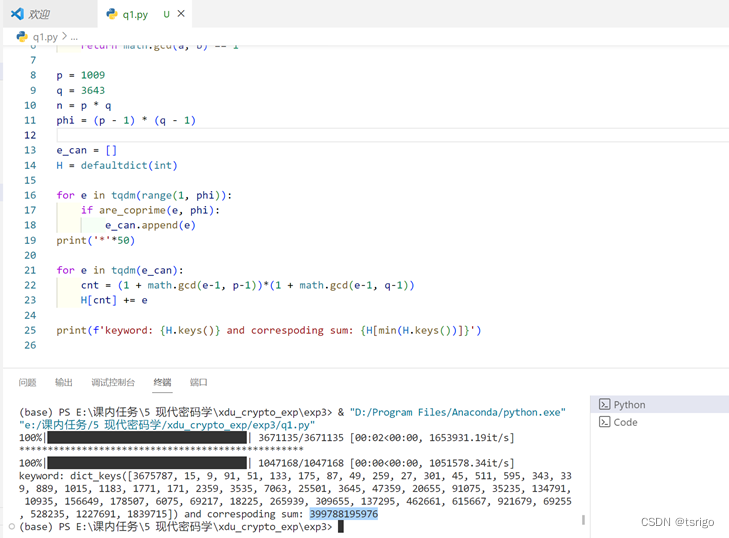
import math
from collections import defaultdict
from tqdm import tqdmdef are_coprime(a, b):return math.gcd(a, b) == 1p = 1009
q = 3643
n = p * q
phi = (p - 1) * (q - 1)e_can = []
H = defaultdict(int)for e in tqdm(range(1, phi)):if are_coprime(e, phi):e_can.append(e)
print('*'*50)for e in tqdm(e_can):cnt = (1 + math.gcd(e-1, p-1))*(1 + math.gcd(e-1, q-1))H[cnt] += eprint(f'keyword: {H.keys()} and correspoding sum: {H[min(H.keys())]}')第二题:
按部就班实现即可,其中求逆元用拓展欧几里得定理。
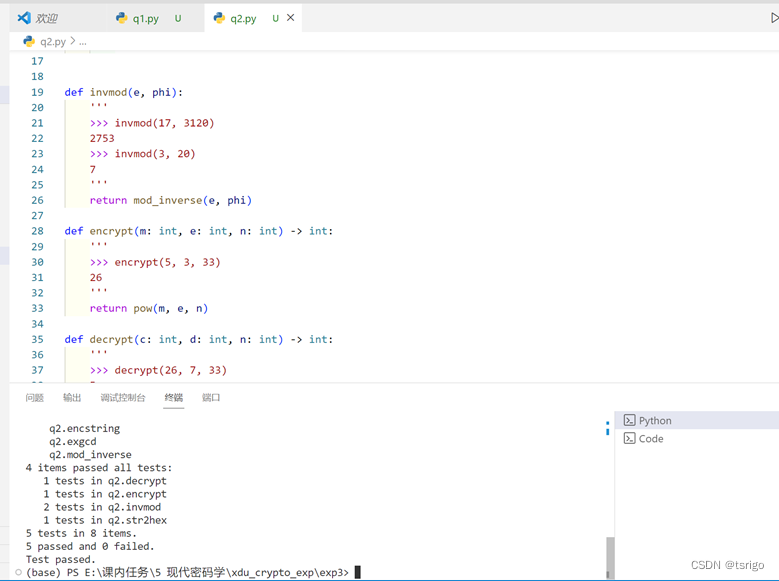
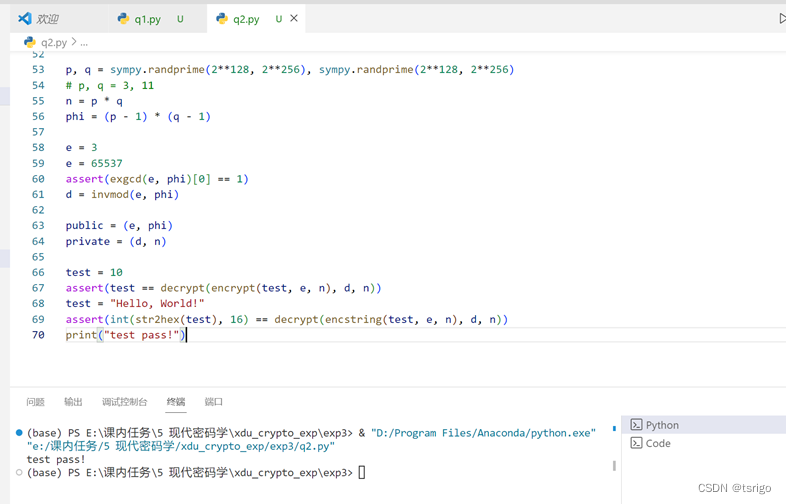
代码和运行结果如下:
import sympy
import binasciidef exgcd(a, b):if a == 0:return b, 0, 1else:gcd, x, y = exgcd(b % a, a)return gcd, y - (b // a) * x, xdef mod_inverse(a, m):gcd, x, _ = exgcd(a, m)if gcd != 1:raise Exception('Modular inverse does not exist')else:return x % mdef invmod(e, phi):'''>>> invmod(17, 3120)2753>>> invmod(3, 20)7'''return mod_inverse(e, phi)def encrypt(m: int, e: int, n: int) -> int: '''>>> encrypt(5, 3, 33)26'''return pow(m, e, n)def decrypt(c: int, d: int, n: int) -> int:'''>>> decrypt(26, 7, 33)5'''return pow(c, d, n)def str2hex(s):'''>>> str2hex("Hello, World!")'48656c6c6f2c20576f726c6421''''return binascii.hexlify(s.encode()).decode()def encstring(m: str, e: int, n: int) -> int:hexstr = str2hex(m)return encrypt(int(hexstr, 16), e, n)p, q = sympy.randprime(2**128, 2**256), sympy.randprime(2**128, 2**256)
# p, q = 3, 11
n = p * q
phi = (p - 1) * (q - 1)e = 3
e = 65537
assert(exgcd(e, phi)[0] == 1)
d = invmod(e, phi)public = (e, phi)
private = (d, n)test = 10
assert(test == decrypt(encrypt(test, e, n), d, n))
test = "Hello, World!"
assert(int(str2hex(test), 16) == decrypt(encstring(test, e, n), d, n))
print("test pass!")


)




)




-Part.01 关于HDP)





)
