根据二叉树创建字符串
本题的关键在于什么情况要省略括号,什么情况不能省略:
- 左右为空可以省略括号
- 左不为空,右为空可以省略括号
- 左为空,右不为空不能省略括号
class Solution {
public://1.左右为空可以省略括号//2.左不为空,右为空可以省略括号//3.左为空,右不为空不能省略括号string tree2str(TreeNode* root) {string ret;if (root == nullptr){return ret;}ret += to_string(root->val);if (root->left || root->right){ret += '(';ret += tree2str(root->left);ret += ')';}if (root->right){ret += '(';ret += tree2str(root->right);ret += ')';}return ret;}
};
二叉树的层序遍历
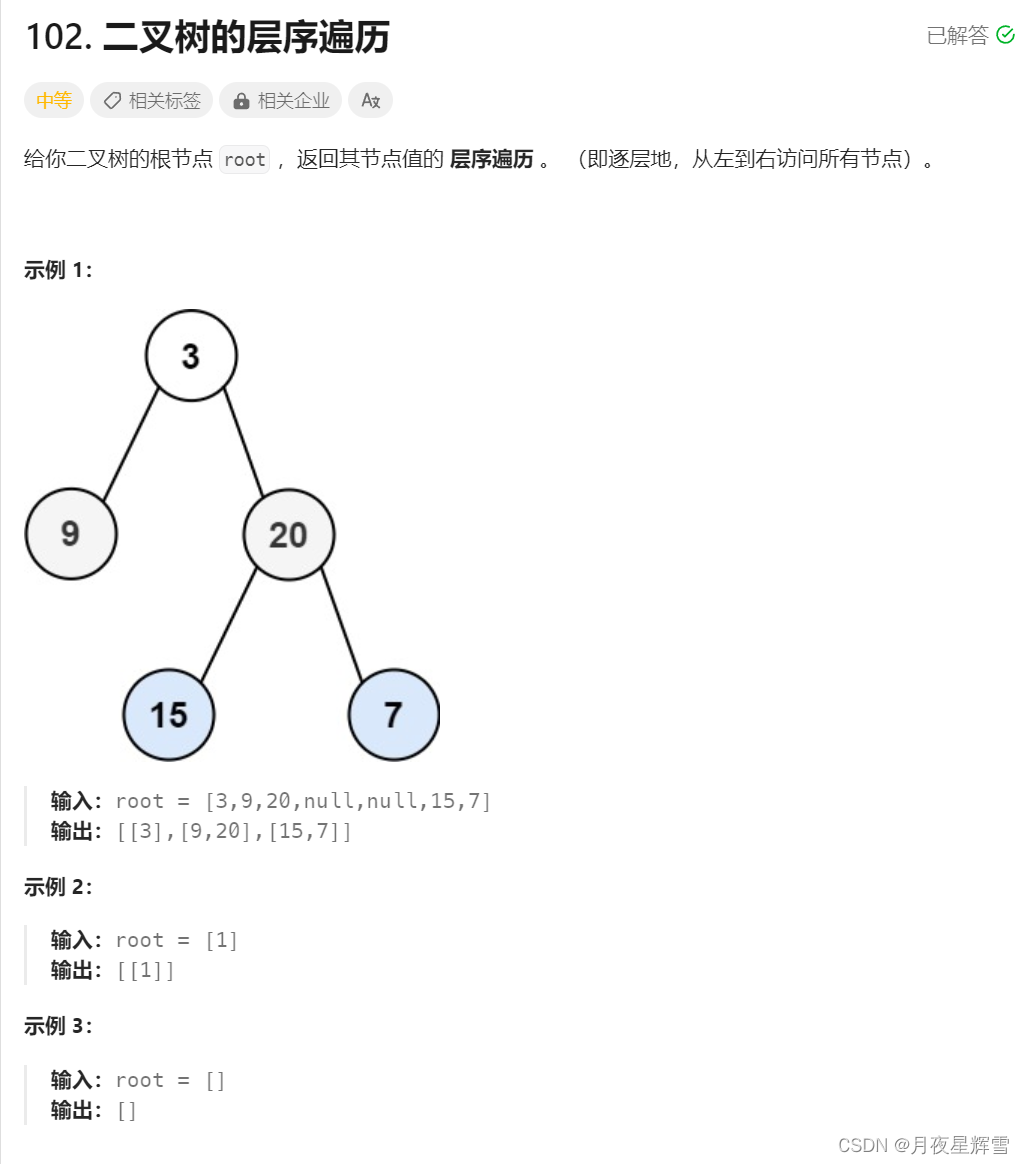
层序遍历咋一看很简单,借助队列就能实现,但是关键在于如何把每一层的结点区分开来,放到不同的vector中。这里采用的方法是使用一个计数器count来记录本层的结点数,count减到0时,本层结点已经遍历完了,此时队列中的就是下一层的结点,用队列的元素个数更新count即可。
class Solution {
public:vector<vector<int>> levelOrder(TreeNode* root) {vector<vector<int>> ret;if (root == nullptr){return ret;}queue<TreeNode*> q;q.push(root);int count = 1;//记录本层的结点数while (!q.empty()){vector<int> v;while (count--){TreeNode* front = q.front();q.pop();v.push_back(front->val);if (front->left){q.push(front->left);}if (front->right){q.push(front->right);}}ret.push_back(v);count = q.size();}return ret;}
};
二叉树的最近公共祖先
思路:找到根结点到p结点和q结点的路径,对比两个路径即可得到答案,时间复杂度O(n)
class Solution {
public:TreeNode* lowestCommonAncestor(TreeNode* root, TreeNode* p, TreeNode* q) {vector<TreeNode*> pPath;vector<TreeNode*> qPath;findPath(root, p, pPath);findPath(root, q, qPath);int minSize = min(pPath.size(), qPath.size());int i = 0;for (; i < minSize; i++){if (pPath[i] != qPath[i]){break;}}return pPath[i - 1];}bool findPath(TreeNode* root, TreeNode* target, vector<TreeNode*>& path){if (root == nullptr){return false;}path.push_back(root);if (root == target){return true;}if (findPath(root->left, target, path)){return true;}if (findPath(root->right, target, path)){return true;}//回溯--恢复现场path.pop_back();return false;}
};二叉搜索树转双向链表

要将二叉搜索树转换为有序的双向链表,那么肯定与中序遍历有关。中序遍历过程中用prev记录上一个遍历的结点,cur记录当前结点,prev->right = cur, cur->left = prev即可。
class Solution {
public:void InOrderConvert(TreeNode* cur, TreeNode*& prev){if (cur == nullptr){return;}InOrderConvert(cur->left, prev);cur->left = prev;if (prev){prev->right = cur;}prev = cur;InOrderConvert(cur->right, prev);}TreeNode* Convert(TreeNode* pcurOfTree) {TreeNode* ret = pcurOfTree;if (ret == nullptr){return ret;}//找到最小的结点--也就是重新链接后双链表的头结点while (ret->left){ret = ret->left;}TreeNode* prev = nullptr;InOrderConvert(pcurOfTree, prev);return ret;}
};
根据前序序列与中序序列构建二叉树

手工构建二叉树很容易,本题难点在于如何将手动构建的过程转化成代码。想一想手工构建的过程。如何确定二叉树的根结点?看前序序列。如何确定左右子树有哪些结点?看中序序列。如何构建左右子树呢? 先确定根节点,这样又转化成了第一个问题。
牢记这一点:前序序列确定根结点。,中序序列确定左右子树有哪些结点。怎么表示左右子树的结点?用一个区间标识范围即可。
class Solution {
public:TreeNode* _buildTree(vector<int>& preorder, vector<int>& inorder, int& prei, int inbegin, int inend){//中序序列区间不存在if (inbegin > inend){return nullptr;}//根据前序序列确定根结点TreeNode* root = new TreeNode(preorder[prei]);prei++;//根据中序序列确定左右子树的结点int pos = inbegin;while (pos <= inend) {if (root->val == inorder[pos]){break;}pos++;}//[inbegin, pos - 1] [pos + 1, inend]root->left = _buildTree(preorder, inorder, prei, inbegin, pos - 1);root->right = _buildTree(preorder, inorder, prei, pos + 1, inend);return root;}TreeNode* buildTree(vector<int>& preorder, vector<int>& inorder) {int prei = 0;return _buildTree(preorder, inorder, prei, 0, inorder.size()- 1);}
};根据后序序列和中序序列构建二叉树
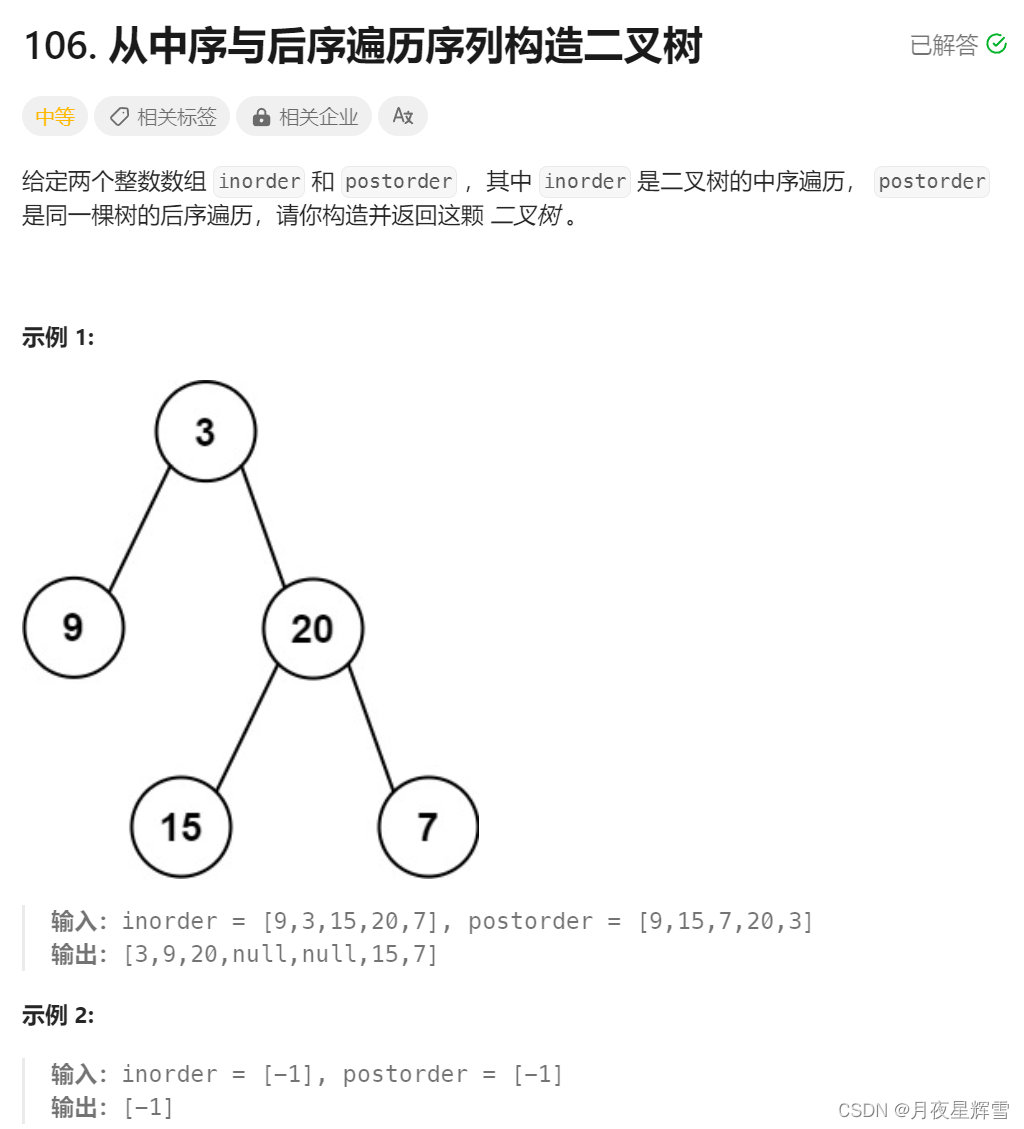
思路和上一题类似,不过要注意postorder要从后往前遍历,并且要先构建右子树,再构建左子树。
class Solution {
public:TreeNode* buildTree(vector<int>& inorder, vector<int>& postorder) {int posti = postorder.size() - 1;return _buildTree(inorder, postorder, posti, 0, inorder.size() - 1);}TreeNode* _buildTree(vector<int>& inorder, vector<int>& postorder, int& posti, int inbegin, int inend){if (inbegin > inend){return nullptr;}//后序序列确定根节点TreeNode* root = new TreeNode(postorder[posti]);posti--;//中序序列确定左右子树int pos = inbegin;while (pos <= inend){if (root->val == inorder[pos]){break;}pos++;}//[inbegin, pos - 1] [pos + 1, inend]//构建右子树和左子树root->right = _buildTree(inorder, postorder, posti, pos + 1, inend);root->left = _buildTree(inorder, postorder, posti, inbegin, pos - 1);return root;}
};二叉树的前序遍历迭代版本
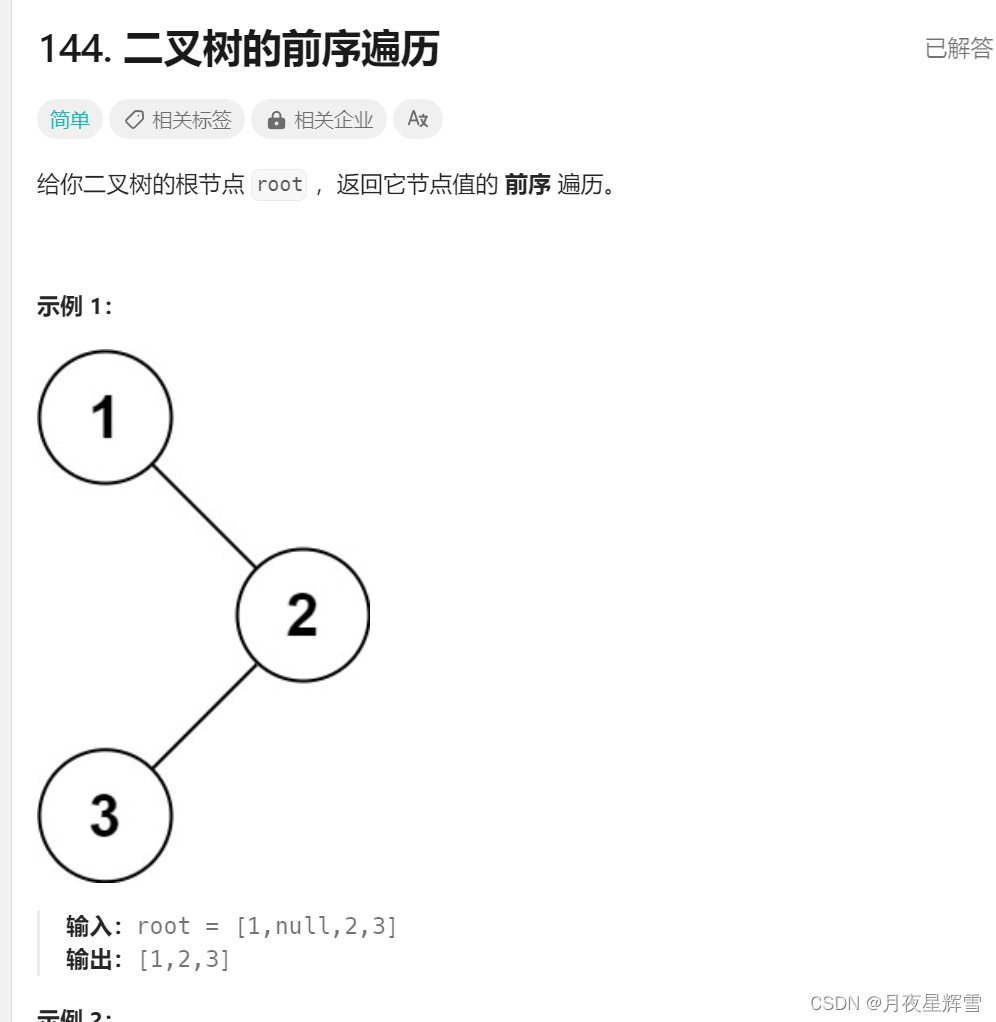
递归写法很简单,如何用迭代来完成呢?
将一颗树分为左路结点和左路结点的右子树,遍历到一颗树的左路结点时就访问它,并将它放入栈中,继续访问下一个左路结点。当左路结点访问完后,从栈中取结点,该结点就是最近的一个左路结点,接下来访问它的右子树,又回到了最开始的步骤。
class Solution {
public:vector<int> preorderTraversal(TreeNode* root) {stack<TreeNode*> st;vector<int> ret;TreeNode* cur = root;while (cur || !st.empty()){//遍历左路结点并访问while (cur){ret.push_back(cur->val);st.push(cur);cur = cur->left;}//遍历左路结点的右子树TreeNode* top = st.top();cur = top->right;st.pop();}return ret;}
};二叉树的中序遍历迭代版本

和上一题类似,一颗树看作左路结点和左路结点的右子树,和前序遍历不同的是,结点的访问时机不同。前序遍历是遍历到某个结点时就要访问它,而中序遍历,遍历到某个结点时先不访问,将它存入栈中,当再把它从栈中取出来时,代表它的左子树已经访问过了,接下来要访问右子树,此时就是访问该结点的时机。
class Solution {
public:vector<int> inorderTraversal(TreeNode* root) {stack<TreeNode*> st;vector<int> ret;TreeNode* cur = root;while (cur || !st.empty()){//遍历左路结点while (cur){st.push(cur);cur = cur->left;}TreeNode* top = st.top();st.pop();//访问左路结点ret.push_back(top->val);//遍历左路结点的右子树cur = top->right;}return ret;}
};二叉树的后序遍历迭代版本

同样,一棵树分为左路结点和左路结点的右子树。左路结点的访问访问时机和前两道题又有所不同。遍历到左路结点时将它存入栈中,不能访问,当它的左子树遍历完时,从栈中取出,此时还是不能访问,要取遍历它的右子树,访问完右子树后,再次从栈中取出,此时才能访问并出栈。
本题难点在于,当你把结点从栈中取出来时,你如何知道它的右子树是否已经访问了呢?此时应该访问这个结点,还是暂时不访问,去遍历它的右子树呢?有两种方案:
1.设置flag值标记结点的右子树是否已经访问。
要让每一个结点都对应一个flag值,怎么做到呢?很简单,再建立一个栈,类型为bool,当结点入栈时,bool类型的变量也跟着入栈(注意不是同一个栈),初始值为false。当从栈中取出结点时,也把对应的bool变量取出,如果为false,说明右子树还没有遍历,将bool变量置为true,访问右子树;若为true,说明右子树已经遍历,访问结点并出栈,同时将bool变量也出栈。结点入栈,bool变量入栈;结点出栈,bool变量也出栈,这样一来就能做到一一对应了。
lass Solution {
public:vector<int> postorderTraversal(TreeNode* root) {stack<TreeNode*> st;stack<bool> flag;vector<int> ret;TreeNode* cur = root;while (cur || !st.empty()){//遍历左路结点while (cur){st.push(cur);flag.push(false);cur = cur->left;}TreeNode* top = st.top();if (top->right == nullptr || flag.top() == true){//访问左路结点ret.push_back(top->val);st.pop();flag.pop();}else{//遍历左路结点的右子树flag.top() = true;cur = top->right;}}return ret;}
};2.标记前一个访问的结点
如果一个结点的右子树 (非空) 遍历完了,那么上一个访问的结点一定是该结点的右孩子。根据这一规律,只需创建一个prev变量来记录上一个访问的结点。如果一个结点从栈中取出来,若该结点右子树为空,则可以直接访问;若该结点右子树不为空且上一个访问的结点是该结点的右孩子,则也可以访问;否则就要先访问该结点的右子树。
class Solution {
public:vector<int> postorderTraversal(TreeNode* root) {vector<int> ret;stack<TreeNode*> st;TreeNode* cur = root;TreeNode* prev = nullptr;while (cur || !st.empty()){//遍历左路结点while (cur){st.push(cur);cur = cur->left;}TreeNode* top = st.top();if (top->right == nullptr || top->right == prev){//访问左路结点ret.push_back(top->val);st.pop();prev = top;}else{//遍历右子树cur = top->right;}}return ret;}
};


,并且在H5中跳转到APP的指定页面)

)






)

使用教程)
)




