做算法课设时候看到题目要求模拟函数递归时候栈的入栈出栈过程。本来想着直接调用系统递归函数即可，可是发现系统函数栈的空间非常小大约只有3000层，很容易爆栈。于是便有了用栈去模拟递归函数的想法，但是上网查了下貌似相关代码比较少，让GPT写的代码又牛头不对马嘴，于是手搓了一下。用栈模拟递归的过程，对递归过程，栈的理解都很有帮助。

二分递归函数模拟

第一个函数erfen为正常的二分递归函数，但是系统的递归栈最多能递归到3000层左右，太少了。我们可以用栈来模拟函数递归的过程，这样栈的层数可以达到我们设置的数组大小，我这里设置了二十万。

#include <iostream>using namespace std;const int N = 2e5 + 5;struct binaryFrame {int l, r;int level;//记录当前层数int step;//记录步数
}stackBinary[N];/*需要模拟的二分递归函数
int erfen(int arr[], int l, int r, int key)
{int ans = -1;int mid = l + r >> 1;if (arr[mid] == key && l == r) return r;if (l >= r) return -1;if (arr[mid] >= key) {ans = erfen(arr, l, mid, key);}else {ans = erfen(arr, mid + 1, r, key);}return ans;
}
*/void binarySearch(int arr[], int l, int r, int key, int& index)
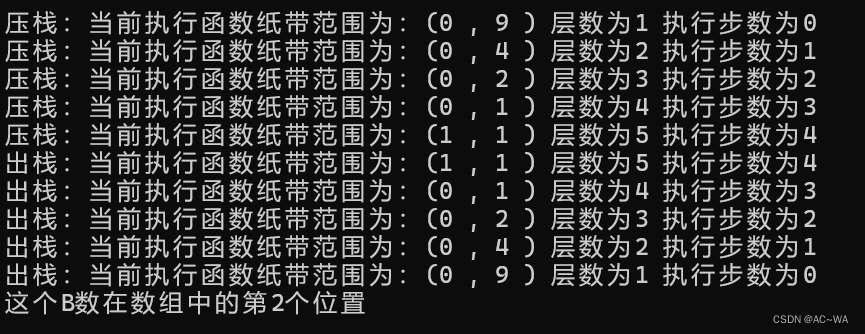
{int nowLevel = 1, nowStep = 0, tt = 0, L = l, R = r;tt++;stackBinary[tt] = { l,r,nowLevel,nowStep };cout << "压栈：" << "当前执行函数纸带范围为：(" << L << " , " << R << " )" << " 层数为" << nowLevel << " 执行步数为" << nowStep << "\n";while (L < R){int mid = L + R >> 1;if (arr[mid] >= key){R = mid;stackBinary[++tt] = { L,R,++nowLevel,++nowStep };cout << "压栈：" << "当前执行函数纸带范围为：(" << L << " , " << R << " )" << " 层数为" << nowLevel << " 执行步数为" << nowStep << "\n";}else {L = mid + 1;stackBinary[++tt] = { L,R,++nowLevel,++nowStep };cout << "压栈：" << "当前执行函数纸带范围为：(" << L << " , " << R << " )" << " 层数为" << nowLevel << " 执行步数为" << nowStep << "\n";}}if (arr[R] == key) {index = R;}while (tt > 0){cout << "出栈：" << "当前执行函数纸带范围为：(" << stackBinary[tt].l << " , " << stackBinary[tt].r << " )" << " 层数为" << stackBinary[tt].level << " 执行步数为" << stackBinary[tt].step << "\n";tt--;}}int main()
{int arr[] = { 1,2,3,4,5,6,7,8,9,10 };int n = 10;int key = 2;int index = -1;binarySearch(arr, 0, n - 1, key, index);if (index == -1) {cout << "找不到这个吊数\n";}else {cout << "这个B数在数组中的第" << index + 1 << "个位置" << "\n";}return 0;
}

显示压栈出栈过程

栈模拟回溯法解决01背包

#include<iostream>
#include<vector>using namespace std;typedef long long LL;const int N = 2e5 + 5;/*
// 全局变量用于存储最终结果
int max_value = 0;
vector<int> best_set;/*
// 需要模拟的递归回溯函数
void knapsack(int index, int current_weight, int current_value, int capacity, const vector<int>& weights, const vector<int>& values, vector<int>& chosen_items) {// 基础情况：如果考虑完所有物品if (index == weights.size()) {// 检查当前物品集合是否是一个有效解if (current_weight <= capacity && current_value > max_value) {max_value = current_value;best_set = chosen_items;}return;}// 选择当前物品chosen_items.push_back(index);knapsack(index + 1, current_weight + weights[index], current_value + values[index], capacity, weights, values, chosen_items);chosen_items.pop_back(); // 回溯// 不选择当前物品knapsack(index + 1, current_weight, current_value, capacity, weights, values, chosen_items);
}*/struct backFrame
{int index;int current_weight;int current_value;int step;vector<int> chosenItems;int stage;
}stackBack[N];void backBag(int n, int capacity, int& max_value, vector<int>& weights, vector<int>& values, vector<int>& chosenItems, vector<int>& best_set)
{int tt = 0, nowStep = 0;stackBack[++tt] = { 0,0,0,0,{},0 };cout << "压栈：初始化压栈，压入 0 0 0 0 {} 0" << "\n";while (tt > 0){backFrame now = stackBack[tt];cout << "当前执行函数层为：" << now.index << " " << now.current_weight << " " << now.current_value << " " << now.step << "\n";if (stackBack[tt].index >= n) {if (stackBack[tt].current_value > max_value && stackBack[tt].current_weight <= capacity){max_value = stackBack[tt].current_value;best_set = stackBack[tt].chosenItems;cout << "更新best_set ";for (auto i : best_set) cout << i + 1 << " ";cout << "\n";}tt--;cout << "出栈1：" << now.index  << " " << now.current_weight<< " " << now.current_value << " " << now.step << "\n";continue;}if (now.stage == 0) {//栈顶为初始状态的话直接下一层函数压栈chosenItems.push_back(now.index);stackBack[++tt] = { now.index + 1,now.current_weight + weights[now.index],now.current_value + values[now.index],now.step + 1,chosenItems,0 };cout << "压栈1: " << now.index + 1 << " " << now.current_weight + weights[now.index] << " " << now.current_value + values[now.index] << " " << now.step + 1 << "\n";stackBack[tt - 1].stage ++;}else if (now.stage == 1) {chosenItems.pop_back();stackBack[++tt] = { now.index + 1,now.current_weight,now.current_value,now.step + 1,chosenItems,0 };cout << "压栈2: " << now.index + 1 << " " << now.current_weight << " " << now.current_value  << " " << now.step + 1 << "\n";stackBack[tt - 1].stage ++;}else if (now.stage == 2) {tt--;cout << "出栈2：" << now.index  << " " << now.current_weight<< " " << now.current_value << " " << now.step << "\n";}}}int main()
{vector<int> weights = { 1,2,3,5,7,6 };vector<int> values = { 6,5,10,30,15,25 };vector<int> chosenItems;vector<int> best_set;int capacity = 10, n = 6;int max_value = -1;/*cout << "请输入物品件数和背包容量\n";cin >> n >> capacity;cout << "请输入" << n << "件物品,每件物品的质量，价值\n";for (int i = 0; i < n; i++){int x, y;cin >> x >> y;weights.push_back(x);values.push_back(y);}*/backBag(n, capacity, max_value, weights, values, chosenItems, best_set);LL sum = 0;for (auto i : best_set){cout << "选择了第" << i + 1 << "件物品\n";sum += weights[i];}cout << "可以获得的最大价值为" << max_value << "\n";cout << "所占用的背包空间为" << sum << "\n";return 0;
}/*
6 10
1 6
2 5
3 10
5 30
7 15
6 25
*/

栈模拟备忘录法解决01背包

#include <iostream>
#include <vector>
#include <cstring>
#include<Windows.h>using namespace std;const int N = 2e4 + 5;int n, W;int dp[505][1005]; // 备忘录数组
vector<int> weights;
vector<int> values;/*需要模拟的备忘录递归函数
// 递归函数，包含备忘录算法逻辑，改为void类型
void knapsack(int idx, int W, int N, int& maxVal) {// 基本情况if (idx == N || W == 0) {maxVal = 0;cout << "边界：" << idx << " " << W << " " << maxVal << "\n";return;}// 检查是否已经计算过if (dp[idx][W] != -1) {maxVal = dp[idx][W];cout << "已经计算过：" << idx << " " << W << " " << maxVal << "\n";return;}// 选择不包含当前物品knapsack(idx + 1, W, N, maxVal);int without = maxVal; // 从上一次递归中获取结果cout << "without:" << idx << " " << W << " " << without << "\n";int with = 0;// 选择包含当前物品，如果背包容量足够if (W >= weight[idx]) {knapsack(idx + 1, W - weight[idx], N, with);with = values[idx] + with; // 添加当前物品的价值cout << "with:" << idx << " " << W << " " << with << "\n";}// 计算最大价值并存储备忘录结果maxVal = max(without, with);dp[idx][W] = maxVal; // 存储备忘录结果//cout<<"idx=,W=,dp= "<<idx<<" "<<W<<" "<<dp[idx]
}*/struct memoFrame
{int index;int with;int without;int max_value;int capacity;int stage;
}stackMemo[N];void memoBag(int index, int capacity, vector<int>& weights, vector<int>& values)
{int tt = 0;stackMemo[++tt] = { 0,0,0,0,capacity,0 };int max_value = 0;while (tt > 0){//cout << "cnt=" << cnt << "\n";memoFrame& now = stackMemo[tt];//cout << "当前函数层为,index,capacity,stage=" << now.index << " " << now.capacity << " " << now.stage << "\n";if ((now.index == n || now.capacity <= 0)) {memoFrame& last = stackMemo[tt - 1];last.max_value = 0;//cout << "当前层为：，返回给上一层的返回值为" << now.index << " " << now.capacity << " " << last.max_value << "\n";tt--;//cout << "出栈：index,capacity,return" << now.index << " " << now.capacity << " " << last.max_value << "\n";continue;}if (dp[now.index][now.capacity] != -1){memoFrame& last = stackMemo[tt - 1];last.max_value = dp[now.index][now.capacity];//cout << "当前层为：，返回给上一层的返回值为" << now.index << " " << now.capacity << " " << last.max_value << "\n";tt--;//cout << "出栈：index,capacity,return" << now.index << " " << now.capacity << " " << last.max_value << "\n";continue;}if (now.stage == 0){stackMemo[++tt] = { now.index + 1,0,0,0,now.capacity,0 };//cout << "压栈0：" << now.index + 1 << " " << now.capacity << " " << "\n";now.stage++;}else if (now.stage == 1){now.without = now.max_value;//cout << "更新without：index, without:" << now.index << " " << now.without << "\n";now.stage++;}else if (now.stage == 2){if (now.capacity >= weights[now.index]) stackMemo[++tt] = { now.index + 1, 0, 0, 0, now.capacity - weights[now.index], 0 };// cout << "压栈1：" << now.index + 1 << " " << now.capacity << " " << "\n";now.stage++;}else if (now.stage == 3){if (now.capacity >= weights[now.index]){now.with = now.max_value + values[now.index];//cout << "更新with, index,with:" << now.index << " " << now.with << "\n";}now.stage++;}else if (now.stage == 4){memoFrame& last = stackMemo[tt - 1];dp[now.index][now.capacity] = max(now.with, now.without);for (int i = 0; i < n; i++){for (int j = 0; j < W; j++){cout << dp[i][j] << " ";}cout << "\n";}last.max_value = dp[now.index][now.capacity];Sleep(1000);//cout << "更新dp: index,dp:" << now.index << " " << last.max_value << "\n";tt--;//cout << "出栈：index,capacity,return" << now.index << " " << now.capacity << " " << last.max_value << "\n";}}}int main() {cin >> n >> W;; // 读取物品数量和背包容量weights.resize(n);values.resize(n);for (int i = 0; i < n; ++i) {cin >> weights[i] >> values[i];}memset(dp, -1, sizeof(dp));memoBag(0, W, weights, values);cout << "最大价值：" << dp[0][W] << endl;return 0;
}/*
6 10
1 6
2 5
3 10
5 30
7 15
6 25
*/

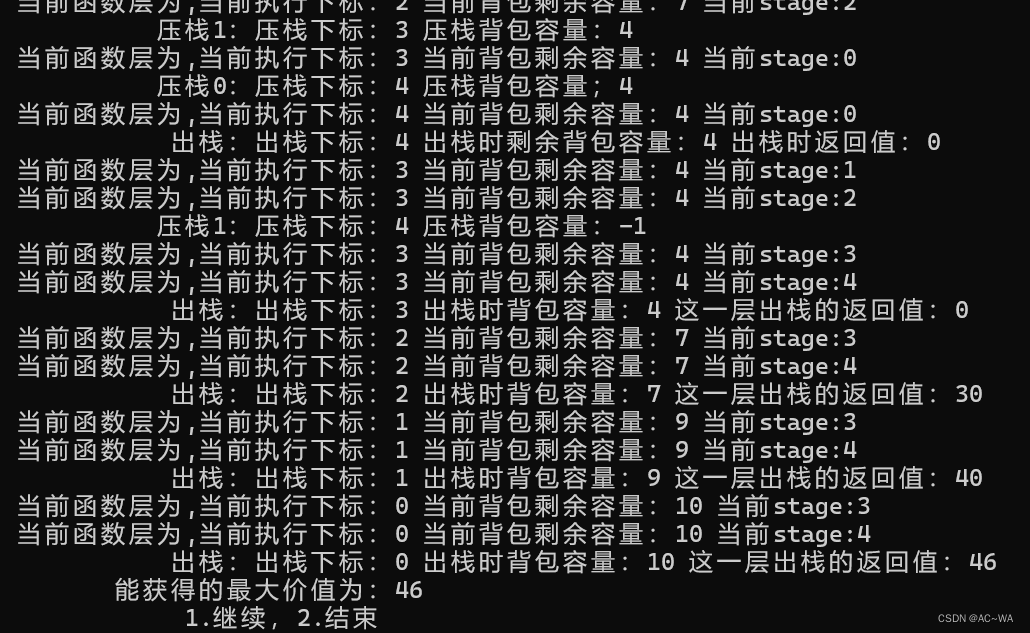
把三个题整合到一块

#include <iostream>
#include <vector>
#include <cstring>
#include<iomanip>
#include <windows.h>using namespace std;const int N = 2e4 + 5;typedef long long LL;int n, W;//n可以是二分的数组长度，也可以是背包的物品个数，W是背包容量int ansPos=-1;//二分搜索到的答案位置int arr[N];int dp[505][1005]; // 备忘录数组
vector<int> weights;
vector<int> values;struct binaryFrame {int l, r;int level;//记录当前层数int step;//记录步数
}stackBinary[N];struct backFrame
{int index;int current_weight;int current_value;int step;vector<int> chosenItems;int stage;
}stackBack[N];struct memoFrame
{int index;int with;int without;int max_value;int capacity;int stage;
}stackMemo[N];void binarySearch(int l, int r, int key)
{int nowLevel = 1, nowStep = 0, tt = 0, L = l, R = r;tt++;stackBinary[tt] = { l,r,nowLevel,nowStep };cout<<right<<setw(50) << "压栈：" << "当前执行函数纸带范围为：(" << L << " , " << R << " )" << " 层数为" << nowLevel << " 执行步数为" << nowStep << "\n";Sleep(200);while (L < R){int mid = L + R >> 1;if (arr[mid] >= key){R = mid;stackBinary[++tt] = { L,R,++nowLevel,++nowStep };cout<<right<<setw(50) << "压栈：" << "当前执行函数纸带范围为：(" << L << " , " << R << " )" << " 层数为" << nowLevel << " 执行步数为" << nowStep << "\n";Sleep(200);}else {L = mid + 1;stackBinary[++tt] = { L,R,++nowLevel,++nowStep };cout<<right<<setw(50) << "压栈：" << "当前执行函数纸带范围为：(" << L << " , " << R << " )" << " 层数为" << nowLevel << " 执行步数为" << nowStep << "\n";Sleep(200);}}if (arr[R] == key) {ansPos = R;}while (tt > 0){cout<<right<<setw(50) << "出栈：" << "当前执行函数纸带范围为：(" << stackBinary[tt].l << " , " << stackBinary[tt].r << " )" << " 层数为" << stackBinary[tt].level << " 执行步数为" << stackBinary[tt].step << "\n";Sleep(200);tt--;}}void backBag(int n, int capacity, int& max_value, vector<int>& weights, vector<int>& values, vector<int>& chosenItems, vector<int>& best_set)
{int tt = 0, nowStep = 0;stackBack[++tt] = { 0,0,0,0,{},0 };cout<<right<<setw(50) << "压栈：初始化压栈，压入 0 0 0 0 {} 0" << "\n";Sleep(200);while (tt > 0){backFrame now = stackBack[tt];cout << right << setw(50) << "当前执行函数层为：下标为：" << now.index << " 当前重量：" << now.current_weight << " 当前价值：" << now.current_value << " 当前步数：" << now.step << "\n";Sleep(200);if (stackBack[tt].index >= n) {if (stackBack[tt].current_value > max_value && stackBack[tt].current_weight <= capacity){max_value = stackBack[tt].current_value;best_set = stackBack[tt].chosenItems;}tt--;cout<<right<<setw(50) << "出栈1：出栈下标：" << now.index << " 当前重量：" << now.current_weight << " 当前价值：" << now.current_value << " 当前步数：" << now.step << "\n";Sleep(200);continue;}if (now.stage == 0) {//栈顶为初始状态的话直接下一层函数压栈chosenItems.push_back(now.index);stackBack[++tt] = { now.index + 1,now.current_weight + weights[now.index],now.current_value + values[now.index],now.step + 1,chosenItems,0 };cout<<right<<setw(50) << "压栈1: 压栈下标：" << now.index + 1 << " 压栈重量：" << now.current_weight + weights[now.index] << " 压栈价值：" << now.current_value + values[now.index] << " 压栈步数：" << now.step + 1 << "\n";Sleep(200);stackBack[tt - 1].stage++;}else if (now.stage == 1) {chosenItems.pop_back();stackBack[++tt] = { now.index + 1,now.current_weight,now.current_value,now.step + 1,chosenItems,0 };cout<<right<<setw(50) << "压栈2: 压栈下标：" << now.index + 1 << " 压栈重量：" << now.current_weight << " " << now.current_value << " " << now.step + 1 << "\n";Sleep(200);stackBack[tt - 1].stage++;}else if (now.stage == 2) {tt--;cout<<right<<setw(50) << "出栈2：" << now.index << " 出栈重量：" << now.current_weight << " 出栈价值：" << now.current_value << " 出栈步数" << now.step << "\n";Sleep(200);}}}void memoBag(int index, int capacity, vector<int>& weights, vector<int>& values)
{int tt = 0;stackMemo[++tt] = { 0,0,0,0,capacity,0 };int max_value = 0;while (tt > 0){memoFrame& now = stackMemo[tt];cout<<right<<setw(50) << "当前函数层为,当前执行下标：" << now.index << " 当前背包剩余容量：" << now.capacity << " 当前stage:" << now.stage << "\n";Sleep(200);if ((now.index == n || now.capacity <= 0)) {memoFrame& last = stackMemo[tt - 1];last.max_value = 0;//cout << "当前层为：，返回给上一层的返回值为" << now.index << " " << now.capacity << " " << last.max_value << "\n";tt--;cout<<right<<setw(50) << "出栈：出栈下标：" << now.index << " 出栈时剩余背包容量：" << now.capacity << " 出栈返回值：" << last.max_value << "\n";Sleep(200);continue;}if (dp[now.index][now.capacity] != -1){memoFrame& last = stackMemo[tt - 1];last.max_value = dp[now.index][now.capacity];//cout << "当前层为：，返回给上一层的返回值为" << now.index << " " << now.capacity << " " << last.max_value << "\n";tt--;cout<<right<<setw(50) << "出栈：出栈下标：" << now.index << " 出栈时剩余背包容量：" << now.capacity << " 出栈时返回值：" << last.max_value << "\n";Sleep(200);continue;}if (now.stage == 0){stackMemo[++tt] = { now.index + 1,0,0,0,now.capacity,0 };cout<<right<<setw(50) << "压栈0：压栈下标：" << now.index + 1 << " 压栈背包容量；" << now.capacity << " " << "\n";Sleep(200);now.stage++;}else if (now.stage == 1){now.without = now.max_value;//cout << "更新without：index, without:" << now.index << " " << now.without << "\n";now.stage++;}else if (now.stage == 2){if (now.capacity >= weights[now.index]) stackMemo[++tt] = { now.index + 1, 0, 0, 0, now.capacity - weights[now.index], 0 };cout<<right<<setw(50) << "压栈1：压栈下标：" << now.index + 1 << " 压栈背包容量：" << now.capacity-weights[now.index] << " " << "\n";Sleep(200);now.stage++;}else if (now.stage == 3){if (now.capacity >= weights[now.index]){now.with = now.max_value + values[now.index];//cout << "更新with, index,with:" << now.index << " " << now.with << "\n";}now.stage++;}else if (now.stage == 4){memoFrame& last = stackMemo[tt - 1];dp[now.index][now.capacity] = max(now.with, now.without);last.max_value = dp[now.index][now.capacity];//cout << "更新dp: index,dp:" << now.index << " " << last.max_value << "\n";tt--;cout<<right<<setw(50) << "出栈：出栈下标：" << now.index << " 出栈时背包容量：" << now.capacity << " 这一层出栈的返回值：" << last.max_value << "\n";Sleep(200);}}}int main() 
{int op;cout<<right<<setw(50) << "请选择要执行的递归函数模拟：1.二分搜索，2.回溯法，3.备忘录法\n";while(cin >> op) {if (op == 1) {int key;cout << right << setw(50) << "栈模拟递归二分算法\n\n\n";cout << right << setw(50) << "请输入查询数组长度\n";cin >> n;cout << right << setw(50) << "请输入" << n << "个数字\n";for (int i = 0; i < n; i++){cin >> arr[i];}cout << right << setw(50) << "请输入需要查找的数字key\n";cin >> key;binarySearch(0, n - 1, key);if (ansPos == -1) cout << right << setw(50) << "找不到这个吊数\n";else cout << right << setw(50) << "数字" << key << "在数组中的第" << ansPos + 1 << "个位置\n";}else if (op == 2){weights.clear();values.clear();cout << right << setw(50) << "栈模拟递归回溯算法\n\n\n";cout << right << setw(50) << "请输入物品件数和背包容量\n";cin >> n >> W;cout << right << setw(50) << "请输入" << n << "件物品的质量和价值\n";for (int i = 0; i < n; i++){int x, y;cin >> x >> y;weights.push_back(x);values.push_back(y);}int max_value = -1;vector<int> best_set;vector<int> chosenItems;backBag(n, W, max_value, weights, values, chosenItems, best_set);cout << right << setw(50) << "可以获得的最大价值为:" << max_value << "\n";for (auto i : best_set){cout << right << setw(50) << "选择了第" << i + 1 << "件物品\n";}}else if (op == 3){weights.clear();values.clear();cout << right << setw(50) << "栈模拟递归备忘录算法\n\n\n";cout << right << setw(50) << "请输入物品件数和背包容量\n";cin >> n >> W;cout << right << setw(50) << "请输入" << n << "件物品的质量和价值\n";for (int i = 0; i < n; i++){int x, y;cin >> x >> y;weights.push_back(x);values.push_back(y);}memset(dp, -1, sizeof(dp));memoBag(0, W, weights, values);cout << right << setw(50) << "能获得的最大价值为：" << dp[0][W] << "\n";}cout << right << setw(50) << "1.继续，2.结束\n";cin >> op;if (op == 2) break;cout << right << setw(50) << "请选择要执行的递归函数模拟：1.二分搜索，2.回溯法，3.备忘录法\n";}return 0;
}/*二分搜索样例输入
10
1 2 3 4 5 6 7 8 9 10
2
*//*回溯与备忘录样例输入
6 10
1 6
2 5
3 10
5 30
7 15
6 25
*//*需要模拟的二分递归函数
int erfen(int arr[], int l, int r, int key)
{int ans = -1;int mid = l + r >> 1;if (arr[mid] == key && l == r) return r;if (l >= r) return -1;if (arr[mid] >= key) {ans = erfen(arr, l, mid, key);}else {ans = erfen(arr, mid + 1, r, key);}return ans;
}
*//*
// 需要模拟的递归回溯函数
void knapsack(int index, int current_weight, int current_value, int capacity, const vector<int>& weights, const vector<int>& values, vector<int>& chosen_items) {// 基础情况：如果考虑完所有物品if (index == weights.size()) {// 检查当前物品集合是否是一个有效解if (current_weight <= capacity && current_value > max_value) {max_value = current_value;best_set = chosen_items;}return;}// 选择当前物品chosen_items.push_back(index);knapsack(index + 1, current_weight + weights[index], current_value + values[index], capacity, weights, values, chosen_items);chosen_items.pop_back(); // 回溯// 不选择当前物品knapsack(index + 1, current_weight, current_value, capacity, weights, values, chosen_items);
}*//*需要模拟的备忘录递归函数
// 递归函数，包含备忘录算法逻辑，改为void类型
void knapsack(int idx, int W, int N, int& maxVal) {// 基本情况if (idx == N || W == 0) {maxVal = 0;cout << "边界：" << idx << " " << W << " " << maxVal << "\n";return;}// 检查是否已经计算过if (dp[idx][W] != -1) {maxVal = dp[idx][W];cout << "已经计算过：" << idx << " " << W << " " << maxVal << "\n";return;}// 选择不包含当前物品knapsack(idx + 1, W, N, maxVal);int without = maxVal; // 从上一次递归中获取结果cout << "without:" << idx << " " << W << " " << without << "\n";int with = 0;// 选择包含当前物品，如果背包容量足够if (W >= weight[idx]) {knapsack(idx + 1, W - weight[idx], N, with);with = values[idx] + with; // 添加当前物品的价值cout << "with:" << idx << " " << W << " " << with << "\n";}// 计算最大价值并存储备忘录结果maxVal = max(without, with);dp[idx][W] = maxVal; // 存储备忘录结果//cout<<"idx=,W=,dp= "<<idx<<" "<<W<<" "<<dp[idx]
}*/