2024年5月19日补充G题。

A - Exponential Plant

Problem Statement

Takahashi is growing a plant. Its height at the time of germination is $0\mathrm{cm}$. Considering the day of germination as day $0$, its height increases by $2^i\mathrm{cm}$ day $i$’s night $(0\le i)$.
Takahashi’s height is $H\mathrm{cm}$.
Every morning, Takahashi measures his height against this plant. Find the first day such that the plant’s height is strictly greater than Takahashi’s height in the morning.

Constraints

$1\le H\le 10^9$
All input values are integers.

Input

The input is given from Standard Input in the following format:

$H$

Output

Print an integer representing the first day such that the plant’s height is greater than Takahashi’s height in the morning.

Sample Input 1

54

Sample Output 1

6

The plant’s height in the mornings of days $1,2,3,4,5,6$ will be $1\mathrm{cm},3\mathrm{cm},7\mathrm{cm},15\mathrm{cm},31\mathrm{cm},63\mathrm{cm}$, respectively. The plant becomes taller than Takahashi in the morning day $6$, so print $6$.

Sample Input 2

7

Sample Output 2

4

The plant’s height will be $7\mathrm{cm}$ in the morning of day $3$ and $15\mathrm{cm}$ in the morning day $4$. The plant becomes taller than Takahashi in the morning of day $4$, so print $4$. Note that, in the morning of day $3$, the plant is as tall as Takahashi, but not taller.

Sample Input 3

262144

Sample Output 3

19

Solution

具体见文末视频。

---

Code

#include <bits/stdc++.h>
#define fi first
#define se second
#define int long longusing namespace std;typedef pair<int, int> PII;
typedef long long LL;signed main() {cin.tie(0);cout.tie(0);ios::sync_with_stdio(0);int x;cin >> x;int j = 1;while ((1ll << j) - 1 <= x) j ++;cout << j << endl;return 0;
}

B - AtCoder Janken 2

Problem Statement

$N$ AtCoder users have gathered to play AtCoder RPS 2. The $i$-th user’s name is $S_i$ and their rating is $C_i$.
AtCoder RPS 2 is played as follows:
Assign the numbers $0,1,\dots ,N-1$ to the users in lexicographical order of their usernames.
Let $T$ be the sum of the ratings of the $N$ users. The user assigned the number $T\mathrm{mod}N$ is the winner.
Print the winner’s username.

What is lexicographical order? Lexicographical order, simply put, means "the order in which words appear in a dictionary." More precisely, the algorithm to determine the order of two distinct strings $S$ and $T$ consisting of lowercase English letters is as follows: Here, "the $i$-th character of $S$" is denoted as $S_i$. If $S$ is lexicographically smaller than $T$, we write $S \lt T$, and if $S$ is larger, we write $S \gt T$.

1. Let $L$ be the length of the shorter string among $S$ and $T$. Check if $S_i$ and $T_i$ match for $i=1,2,\dots,L$. If there exists an $i$ such that $S_i \neq T_i$, let $j$ be the smallest such $i$. Compare $S_j$ and $T_j$. If $S_j$ is alphabetically smaller than $T_j$, then $S \lt T$. Otherwise, $S \gt T$. The algorithm ends here. If there is no $i$ such that $S_i \neq T_i$, compare the lengths of $S$ and $T$. If $S$ is shorter than $T$, then $S \lt T$. If $S$ is longer, then $S \gt T$. The algorithm ends here.

## Constraints

$1\le N\le 100$
$S_i$ is a string consisting of lowercase English letters with length between $3$ and $16$, inclusive.
$S_1,S_2,\dots ,S_N$ are all distinct.
$1\le C_i\le 4229$
$C_i$ is an integer.

Input

The input is given from Standard Input in the following format:

$N$
$S_1$ $C_1$
$S_2$ $C_2$
$⋮$
$S_N$ $C_N$

Output

Print the answer on a single line.

Sample Input 1

3
takahashi 2
aoki 6
snuke 5

Sample Output 1

snuke

The sum of the ratings of the three users is $13$. Sorting their names in lexicographical order yields aoki, snuke, takahashi, so aoki is assigned number $0$, snuke is $1$, and takahashi is $2$.
Since $13\mathrm{mod}3=1$, print snuke, who is assigned number $1$.

Sample Input 2

3
takahashi 2813
takahashixx 1086
takahashix 4229

Sample Output 2

takahashix

Solution

具体见文末视频。

Code

#include <bits/stdc++.h>
#define fi first
#define se second
#define int long longusing namespace std;typedef pair<int, int> PII;
typedef long long LL;signed main() {cin.tie(0);cout.tie(0);ios::sync_with_stdio(0);int n;cin >> n;std::vector<string> s(n);int x, sum = 0;for (int i = 0; i < n; i ++)cin >> s[i] >> x, sum += x;sort(s.begin(), s.end());cout << s[sum % n] << endl;return 0;
}

C - AtCoder Magics

Problem Statement

Takahashi has $N$ cards from the card game “AtCoder Magics.” The $i$-th card will be called card $i$. Each card has two parameters: strength and cost. Card $i$ has a strength of $A_i$ and a cost of $C_i$.
He does not like weak cards, so he will discard them. Specifically, he will repeat the following operation until it can no longer be performed:
Choose two cards $x$ and $y$ such that KaTeX parse error: Expected 'EOF', got '&' at position 5: A_x &̲gt; A_y and KaTeX parse error: Expected 'EOF', got '&' at position 5: C_x &̲lt; C_y. Discard card $y$.
It can be proved that the set of remaining cards when the operations can no longer be performed is uniquely determined. Find this set of cards.

Constraints

$2\le N\le 2\times 10^5$
$1\le A_i,C_i\le 10^9$
$A_1,A_2,\dots ,A_N$ are all distinct.
$C_1,C_2,\dots ,C_N$ are all distinct.
All input values are integers.

Input

The input is given from Standard Input in the following format:

$N$
$A_1$ $C_1$
$A_2$ $C_2$
$⋮$
$A_N$ $C_N$

Output

Let there be $m$ remaining cards, cards $i_1,i_2,\dots ,i_m$, in ascending order. Print these in the following format:

$m$
$i_1$ $i_2$ $\cdots$ $i_m$

Sample Input 1

3
2 4
1 1
3 2

Sample Output 1

2
2 3

Focusing on cards $1$ and $3$, we have KaTeX parse error: Expected 'EOF', got '&' at position 5: A_1 &̲lt; A_3 and KaTeX parse error: Expected 'EOF', got '&' at position 5: C_1 &̲gt; C_3, so card $1$ can be discarded.
No further operations can be performed. At this point, cards $2$ and $3$ remain, so print them.

Sample Input 2

5
1 1
10 2
100 3
1000 4
10000 5

Sample Output 2

5
1 2 3 4 5

In this case, no cards can be discarded.

Sample Input 3

6
32 101
65 78
2 29
46 55
103 130
52 40

Sample Output 3

4
2 3 5 6

Solution

具体见文末视频。

---

Code

#include <bits/stdc++.h>
#define fi first
#define se second
#define int long longusing namespace std;typedef pair<int, int> PII;
typedef long long LL;const int N = 2e5 + 10;int n;
struct Inf {int a, b, id;bool operator< (const Inf &tmp)const {return a < tmp.a;}
}card[N];signed main() {cin.tie(0);cout.tie(0);ios::sync_with_stdio(0);cin >> n;for (int i = 1; i <= n; i ++)cin >> card[i].a >> card[i].b, card[i].id = i;sort(card + 1, card + 1 + n);set<PII> tmp;for (int i = 1; i <= n; i ++) {while (tmp.size() && (*tmp.rbegin()).fi > card[i].b) tmp.erase(-- tmp.end());tmp.insert({card[i].b, card[i].id});}std::vector<int> res;for (auto v : tmp)res.emplace_back(v.se);sort(res.begin(), res.end());cout << res.size() << endl;for (auto v : res)cout << v << " ";return 0;
}

D - AtCoder Wallpaper

Problem Statement

The pattern of AtCoder’s wallpaper can be represented on the $xy$-plane as follows:
The plane is divided by the following three types of lines:
$x=n$ (where $n$ is an integer)
$y=n$ (where $n$ is an even number)
$x+y=n$ (where $n$ is an even number)
Each region is painted black or white. Any two regions adjacent along one of these lines are painted in different colors.
The region containing $(0.5,0.5)$ is painted black.
The following figure shows a part of the pattern.

You are given integers $A,B,C,D$. Consider a rectangle whose sides are parallel to the $x$- and $y$-axes, with its bottom-left vertex at $(A,B)$ and its top-right vertex at $(C,D)$. Calculate the area of the regions painted black inside this rectangle, and print twice that area.
It can be proved that the output value will be an integer.

Constraints

$-10^9\le A,B,C,D\le 10^9$
KaTeX parse error: Expected 'EOF', got '&' at position 3: A &̲lt; C and KaTeX parse error: Expected 'EOF', got '&' at position 3: B &̲lt; D.
All input values are integers.

Input

The input is given from Standard Input in the following format:

$A$ $B$ $C$ $D$

Output

Print the answer on a single line.

Sample Input 1

0 0 3 3

Sample Output 1

10

We are to find the area of the black-painted region inside the following square:

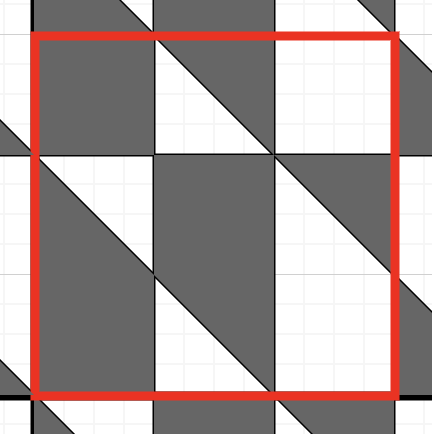
The area is $5$, so print twice that value: $10$.

Sample Input 2

-1 -2 1 3

Sample Output 2

11

The area is $5.5$, which is not an integer, but the output value is an integer.

Sample Input 3

-1000000000 -1000000000 1000000000 1000000000

Sample Output 3

4000000000000000000

This is the case with the largest rectangle, where the output still fits into a 64-bit signed integer.

Solution

具体见文末视频。

---

Code

#include <bits/stdc++.h>
#define fi first
#define se second
#define int long longusing namespace std;typedef pair<int, int> PII;
typedef long long LL;const int INF = 1e9 + 2;int work(int x, int y) {int res = 0;res += (((y + INF) / 2 + 1) / 2 * 2 + ((y + INF) / 2 - ((y + INF) / 2 + 1) / 2) * 6) * ((x + INF) / 2);if (y & 1) {if (((y + INF) / 2) & 1) res += ((x + INF) / 2) * 3;else res += (x + INF) / 2;}if (x & 1) {res += (((y + INF) / 2 + 1) / 2 + ((y + INF) / 2 - ((y + INF) / 2 + 1) / 2) * 3);}if ((x & 1) && (y & 1)) {if (((y + INF) / 2) & 1) {if ((x & 1) ^ (y & 1)) res += 1;else res += 2;} else {if ((x & 1) ^ (y & 1)) res += 1;}}return res;
}signed main() {cin.tie(0);cout.tie(0);ios::sync_with_stdio(0);int a, b, c, d;cin >> a >> b >> c >> d;swap(a, b), swap(c, d);cout << work(c, d) - work(a, d) - work(c, b) + work(a, b) << endl;return 0;
}

---

E - Remove Pairs

Problem Statement

Takahashi and Aoki are playing a game using $N$ cards. The front side of the $i$-th card has $A_i$ written on it, and the back side has $B_i$ written on it. Initially, the $N$ cards are laid out on the table. With Takahashi going first, the two players take turns performing the following operation:
Choose a pair of cards from the table such that either the numbers on their front sides are the same or the numbers on their back sides are the same, and remove these two cards from the table. If no such pair of cards exists, the player cannot perform the operation.
The player who is first to be unable to perform the operation loses, and the other player wins.
Determine who wins if both players play optimally.

Constraints

$1\le N\le 18$
$1\le A_i,B_i\le 10^9$
All input values are integers.

Input

The input is given from Standard Input in the following format:

$N$
$A_1$ $B_1$
$A_2$ $B_2$
$⋮$
$A_N$ $B_N$

Output

Print Takahashi if Takahashi wins when both players play optimally, and Aoki otherwise.

Sample Input 1

5
1 9
2 5
4 9
1 4
2 5

Sample Output 1

Aoki

If Takahashi first removes
the first and third cards: Aoki can win by removing the second and fifth cards.
the first and fourth cards: Aoki can win by removing the second and fifth cards.
the second and fifth cards: Aoki can win by removing the first and third cards.
These are the only three pairs of cards Takahashi can remove in his first move, and Aoki can win in all cases. Therefore, the answer is Aoki.

Sample Input 2

9
3 2
1 7
4 1
1 8
5 2
9 8
2 1
6 8
5 2

Sample Output 2

Takahashi

Solution

具体见文末视频。

---

Code

#include <bits/stdc++.h>
#define fi first
#define se second
#define int long longusing namespace std;typedef pair<int, int> PII;
typedef long long LL;const int N = 20;int n;
int a[N], b[N];
int f[1 << N];bool check(int x) {for (int i = 0; i < n; i ++)for (int j = i + 1; j < n; j ++)if ((x >> i & 1) && (x >> j & 1) && (a[i + 1] == a[j + 1] || b[i + 1] == b[j + 1]))return 0;return 1;
}
int rev(int x) {if (x == 1) return 2;else return 1;
}signed main() {cin.tie(0);cout.tie(0);ios::sync_with_stdio(0);cin >> n;for (int i = 1; i <= n; i ++)cin >> a[i] >> b[i];for (int i = 0; i < 1 << n; i ++)if (check(i))f[i] = 2;for (int i = 0; i < 1 << n; i ++) {for (int j = 0; j < n; j ++)for (int k = j + 1; k < n; k ++)if (!(i >> j & 1) && !(i >> k & 1) && (a[j + 1] == a[k + 1] || b[j + 1] == b[k + 1])) {if (!f[i | (1 << j) | (1 << k)]) f[i | (1 << j) | (1 << k)] = rev(f[i]);else if (f[i | (1 << j) | (1 << k)] == 1) continue;else {if (rev(f[i]) == 1) f[i | (1 << j) | (1 << k)] = 1;}}}if (f[(1 << n) - 1] == 1) cout << "Takahashi" << endl;else cout << "Aoki" << endl;return 0;
}

F - Useless for LIS

Problem Statement

You are given an integer sequence $A$ of length $N$.
For each $t=1,2,\dots ,N$, determine whether $A_t$ is included in a longest increasing subsequence of $A$.
Here, $A_t$ is included in a longest increasing subsequence of $A$ if and only if the following holds:
Let $L$ be the length of a longest increasing subsequence of $A$. There exists a strictly increasing integer sequence $i=(i_1,i_2,\dots ,i_L)(i_1<i_2<\cdots <i_L)$, where each element is between $1$ and $N$, inclusive, that satisfies all of the following conditions:
$A_{i_1}<A_{i_2}<\cdots <A_{i_L}$.
$i_k=t$ for some $k(1\le k\le L)$.
You are given $T$ test cases; solve each of them.

What is a longest increasing subsequence? A subsequence of a sequence $A$ is a sequence that can be derived by extracting some elements from $A$ without changing the order. A longest increasing subsequence of a sequence $A$ is a subsequence of $A$ that is strictly increasing with the greatest possible length. ## Constraints

$1\le T\le 2\times 10^5$
$1\le N\le 2\times 10^5$
$1\le A_i\le 10^9$
The sum of $N$ across all test cases is at most $2\times 10^5$.

Input

The input is given from Standard Input in the following format:

$T$
${\mathrm{case}}_1$
${\mathrm{case}}_2$
$⋮$
${\mathrm{case}}_T$

Here, $\mathrm{cas}{\mathrm{e}}_{\mathrm{i}}$ represents the input for the $i$-th case. Each case is given in the following format:

$N$
$A_1$ $A_2$ $\cdots$ $A_N$

Output

Print the answers in the following format:

${\mathrm{answer}}_1$
${\mathrm{answer}}_2$
$⋮$
${\mathrm{answer}}_T$

Here, ${\mathrm{answer}}_i$ represents the output for the $i$-th case. For each case, let there be $m$ indices $t$ such that $A_t$ is included in a longest increasing subsequence of $A$, which are $i_1,i_2,\dots ,i_m$ in ascending order. Print these in the following format:

$m$
$i_1$ $i_2$ $\cdots$ $i_m$

Sample Input 1

1
5
2 1 4 5 3

Sample Output 1

4
1 2 3 4

One of the longest increasing subsequences is $(2,4,5)$, with a length of $3$. Another longest increasing subsequence is $(1,4,5)$. However, no longest increasing subsequence includes $A_5$.
Therefore, print $1,2,3,4$.

Sample Input 2

2
6
2 5 3 4 3 4
5
10000 1000 100 1 10

Sample Output 2

5
1 3 4 5 6
2
4 5

Solution

具体见文末视频。

---

Code

#include <bits/stdc++.h>
#define fi first
#define se second
#define int long longusing namespace std;typedef pair<int, int> PII;
typedef long long LL;const int N = 2e5 + 10;int n;
int a[N], f[N], g[N];
std::vector<int> dct;
struct fenwick {int tr[N];void add(int x, int d) {for (int i = x; i < N; i += (i & -i)) tr[i] = max(d, tr[i]);}int mx(int x) {int res = 0;if (!x) return res;for (int i = x; i; i -= (i & -i)) res = max(tr[i], res);return res;}
}cnt1, cnt2;int find(int x) {return lower_bound(dct.begin(), dct.end(), x) - dct.begin() + 1;
}void solve() {dct.clear();cin >> n;for (int i = 1; i <= n; i ++)f[i] = g[i] = cnt1.tr[i] = cnt2.tr[i] = 0;for (int i = 1; i <= n; i ++)cin >> a[i], dct.emplace_back(a[i]);sort(dct.begin(), dct.end());dct.erase(unique(dct.begin(), dct.end()), dct.end());for (int i = 1; i <= n; i ++) {f[i] = max(1ll, cnt1.mx(find(a[i]) - 1) + 1);cnt1.add(find(a[i]), f[i]);}for (int i = n; i >= 1; i --) {g[i] = max(1ll, cnt2.mx(dct.size() - find(a[i])) + 1);cnt2.add(dct.size() - find(a[i]) + 1, g[i]);}int ans = 0;for (int i = 1; i <= n; i ++)ans = max(ans, f[i]);std::vector<int> res;for (int i = 1; i <= n; i ++)if (f[i] + g[i] - 1 == ans)res.emplace_back(i);cout << res.size() << endl;for (auto v : res)cout << v << " ";cout << endl;
}signed main() {cin.tie(0);cout.tie(0);ios::sync_with_stdio(0);int dt;cin >> dt;while (dt --)solve();return 0;
}

---

G - Select Strings

Problem Statement

You are given $N$ strings $S_1,S_2,\dots ,S_N$ consisting of lowercase English letters and $N$ positive integers $A_1,A_2,\dots ,A_N$.
A subset $T$ of { 1 , 2 , … , N } \lbrace 1, 2, \ldots, N \rbrace {1,2,…,N} is called a good set if there is no pair $i,j\in T(i\ne j)$ such that $S_i$ is a substring of $S_j$.
Find the maximum possible value of $\sum _{i\in T}{A}_i$ for a good set $T$.

What is a substring? A substring of a string $S$ is a string obtained by deleting zero or more characters from the beginning and zero or more characters from the end of $S$. For example, ab is a substring of abc, but ac is not a substring of abc. ## Constraints

$1\le N\le 100$
$S_i$ is a string consisting of lowercase English letters.
$1\le |S_i|$
$|S_1|+|S_2|+\dots +|S_N|\le 5000$
$1\le A_i\le 10^9$

Input

The input is given from Standard Input in the following format:

$N$
$S_1$
$S_2$
$⋮$
$S_N$
$A_1$ $A_2$ $\dots$ $A_N$

Output

Print the answer.

Sample Input 1

4
atcoder
at
coder
code
5 2 3 4

Sample Output 1

6

The possible good sets $T$ and their corresponding $\sum _{i\in T}{A}_i$ are as follows:
T = { 1 } T = \lbrace 1 \rbrace T={1}: $\sum _{i\in T}{A}_i=5$
T = { 2 } T = \lbrace 2 \rbrace T={2}: $\sum _{i\in T}{A}_i=2$
T = { 3 } T = \lbrace 3 \rbrace T={3}: $\sum _{i\in T}{A}_i=3$
T = { 4 } T = \lbrace 4 \rbrace T={4}: $\sum _{i\in T}{A}_i=4$
T = { 2 , 3 } T = \lbrace 2, 3 \rbrace T={2,3}: $\sum _{i\in T}{A}_i=5$
T = { 2 , 4 } T = \lbrace 2, 4 \rbrace T={2,4}: $\sum _{i\in T}{A}_i=6$
The maximum among them is $6$, so print $6$.

Sample Input 2

10
abcd
abc
ab
a
b
c
d
ab
bc
cd
100 10 50 30 60 90 80 70 40 20

Sample Output 2

260

Solution

G题讲解

---

Code

#include <bits/stdc++.h>
#define fi first
#define se second
#define int long longusing namespace std;typedef pair<int, int> PII;
typedef long long LL;const int N = 2e2 + 10, M = 8e4 + 10, INF = 1e18;int n, s, t;
string S[N];
int a[N], h[N], e[M], ne[M], f[M], idx;
int d[N], cur[N], din[N], dout[N];void add(int a, int b, int c) {e[idx] = b, ne[idx] = h[a], f[idx] = c, h[a] = idx ++;e[idx] = a, ne[idx] = h[b], f[idx] = 0, h[b] = idx ++;
}
int bfs() {memset(d, -1, sizeof d);queue<int> q;q.emplace(s), d[s] = 0, cur[s] = h[s];while (q.size()) {int u = q.front();q.pop();for (int i = h[u]; ~i; i = ne[i]) {int j = e[i];if (d[j] == -1 && f[i]) {d[j] = d[u] + 1, cur[j] = h[j];if (j == t) return 1;q.emplace(j);}}}return 0;
}
int find(int u, int lim) {if (u == t) return lim;int flow = 0;for (int i = cur[u]; ~i && flow < lim; i = ne[i]) {cur[u] = i;int j = e[i];if (d[j] == d[u] + 1 && f[i]) {int tmp = find(j, min(lim - flow, f[i]));if (!tmp) d[j] = -1;f[i] -= tmp, f[i ^ 1] += tmp, flow += tmp;}}return flow;
}
int dinic() {int res = 0, flow;while (bfs()) while (flow = find(s, INF)) res += flow;return res;
}
bool check(string a, string b) {if (a.size() > b.size()) return 0;for (int j = 0; j < b.size() - a.size() + 1; j ++)if (b.substr(j, a.size()) == a)return 1;return 0;
}signed main() {cin.tie(0);cout.tie(0);ios::sync_with_stdio(0);cin >> n;memset(h, -1, sizeof h);s = 0, t = 2 * n + 1;for (int i = 1; i <= n; i ++)cin >> S[i];int sum = 0;for (int i = 1; i <= n; i ++)cin >> a[i], sum += a[i];for (int i = 1; i <= n; i ++)for (int j = 1; j <= n; j ++)if (i != j && check(S[i], S[j]) && (S[i] != S[j] || i < j))add(i, j + n, INF);for (int i = 1; i <= n; i ++)add(s, i, a[i]), add(i + n, t, a[i]);cout << sum - dinic() << endl;return 0;
}

---

视频题解

以下是A到F题视频讲解

---

最后祝大家早日