

前言
AVL树就是在二叉搜索树的基础上引入了平衡因子,因此AVL树也可以看成是二叉搜索树.一棵AVL树或者是空树,或者是具有以下性质的二叉搜索树:它的左右子树都是AVL树,左右子树高度之差(简称平衡因子)的绝对值不超过1(-1/0/1).接下来我们继续学习AVL树底层实现的部分机制.
文章目录
- 前言
- 1.AVL树结构
- 2.AVL树的插入
- ✨左单旋
- ✨右单旋
- ✨右左双旋
- ✨左右双旋
- 3.中序遍历
- 4. AVL树的验证
- 5.验证用例
- 6.结语
1.AVL树结构
//AVL树节点类
template<class K, class V>
struct AVLTreeNode
{pair<K, V> _kv;AVLTreeNode<K, V>* _pLeft;AVLTreeNode<K, V>* _pRight;AVLTreeNode<K, V>* _pParent;int _bf; // balance factorAVLTreeNode(const pair<K, V>& kv):_kv(kv), _pLeft(nullptr), _pRight(nullptr), _pParent(nullptr), _bf(0){}
};// AVL: 二叉搜索树 + 平衡因子的限制
template<class K,class V>
class AVLTree
{typedef AVLTreeNode<K,V> Node;
public:AVLTree(): _pRoot(nullptr){}// 在AVL树中插入值为kv的节点bool Insert(const pair<K, V>& kv);//中序遍历void InOrder(){_InOrder(_pRoot);}//判断是否是平衡树bool IsBalanceTree(){//嵌套一层函数return _IsBalanceTree(_pRoot);}private:bool _IsBalanceTree(Node* pRoot);int _Height(Node* pRoot);void _InOrder(Node* root);// 右单旋void RotateR(Node* parent);// 左单旋void RotateL(Node* parent);// 右左双旋void RotateRL(Node* parent);// 左右双旋void RotateLR(Node* parent)
2.AVL树的插入
AVL树的插入过程可以分为两步:
- 按照二叉搜索树的方式插入新节点
- 调整节点的平衡因子
在插入新节点之后,AVL树的平衡性可能会遭到破坏,此时就需要更新平衡因子,并检测是否破坏了AVL树的平衡性。
如下图所示:
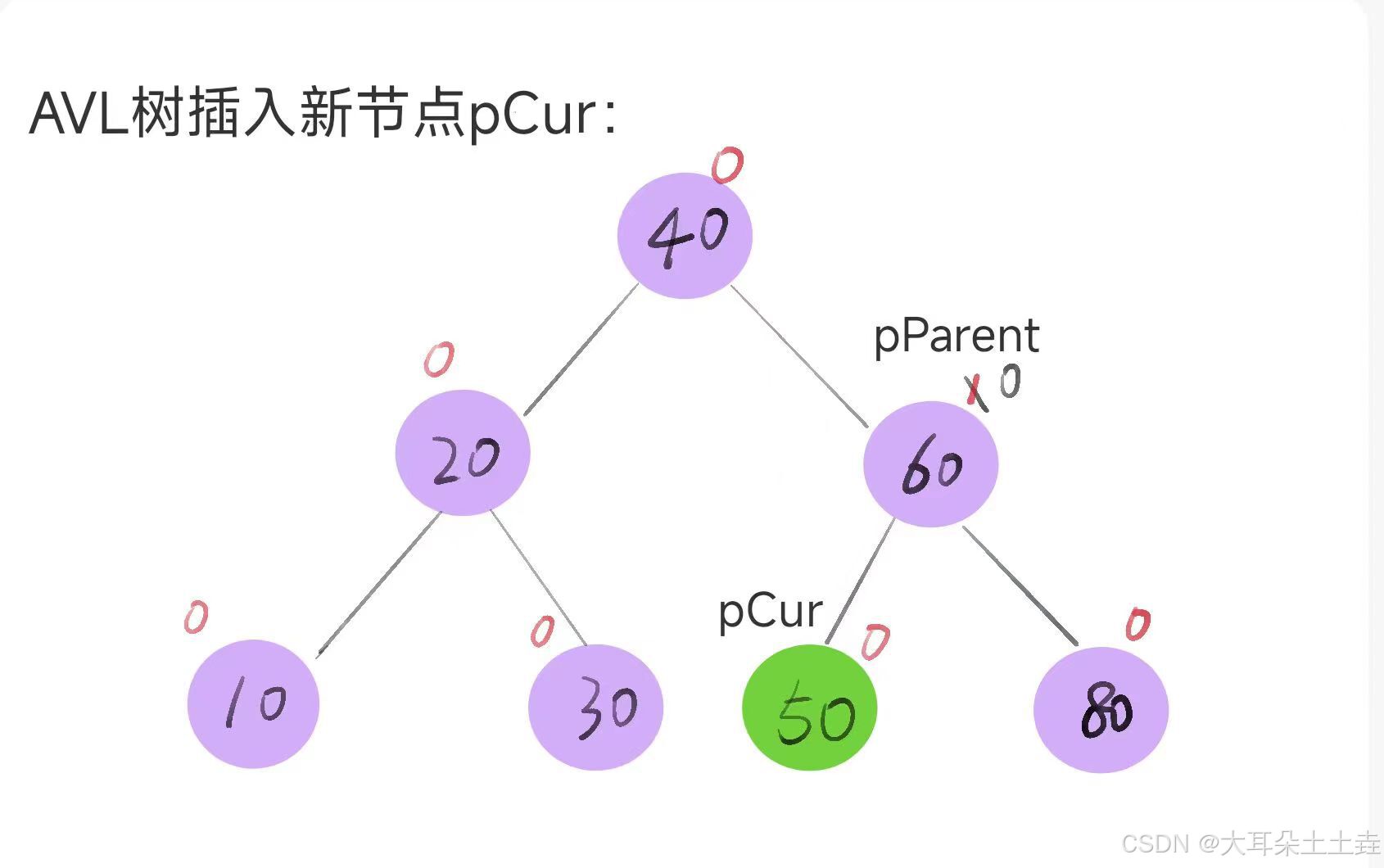
pCur插入后,pParent的平衡因子一定需要调整,在插入之前,pParent
的平衡因子分为三种情况:-1,0, 1, 分以下两种情况:
- 如果pCur插入到pParent的左侧,只需给pParent的平衡因子-1即可,如上图所示
- 如果pCur插入到pParent的右侧,只需给pParent的平衡因子+1即可
此时,pParent的平衡因子也可能有三种情况:0,正负1, 正负2
- 如果pParent的平衡因子为0,说明插入之前pParent的平衡因子为正负1,插入后被调整成0,此时满足AVL树的性质,插入成功,如上图所示;
- 如果pParent的平衡因子为正负1,说明插入前pParent的平衡因子一定为0(不可能是2,因为这样没插入新节点前该树就已经不平衡了),插入后被更新成正负1,此时以pParent为根的树的高度增加,需要继续向上更新,如下图所示:
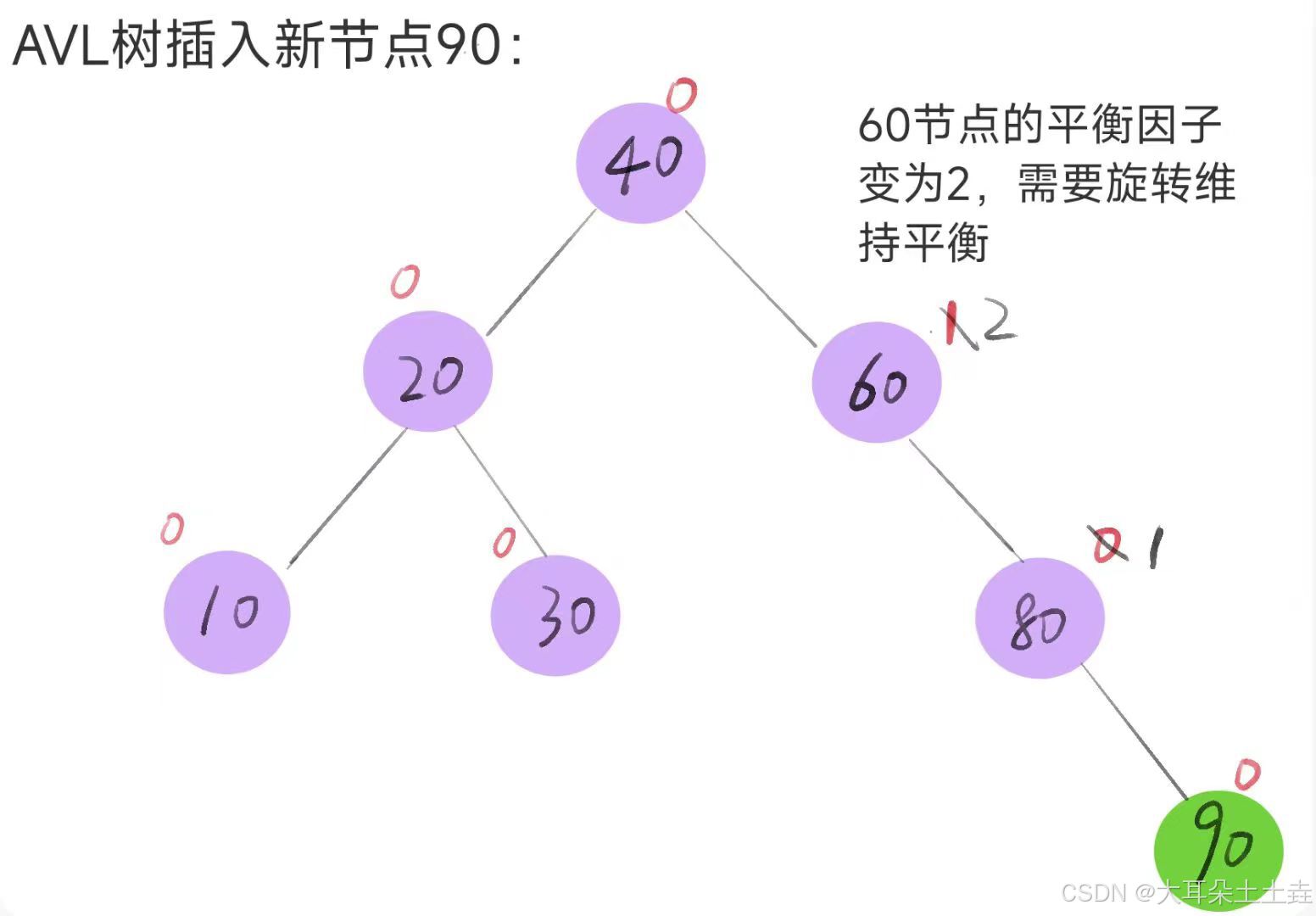
AVL树插入新节点90之后,pParent也就是80节点的平衡因子就需要更新为1,继续往上更新,直到60节点的平衡因子被更新为2,说明不符合AVL树的性质,就需要进行旋转来维持平衡。
- 如果更新后pParent的平衡因子为正负2,则pParent的平衡因子违反平衡树的性质,需要对其进行旋转处理,如上图所示
所以对于AVL树插入新节点来说,我们需要更新插入后由于左右子树高度差改变带来的新的平衡因子,然后根据平衡因子是否大于1或小于-1来判断AVL树是否平衡,如果不平衡我们就必须通过旋转来维持平衡,代码如下:
// 在AVL树中插入值为kv的节点bool Insert(const pair<K, V>& kv){//1.先构造新节点Node* newnode = new Node(kv);Node* cur = _pRoot;//2.判断插入位置if (cur == nullptr){//如果树为空_pRoot = newnode;return true;}//如果AVL树不为空,找到插入位置Node* parent = cur->_pParent;while (cur){ if (cur->_kv.first > kv.first){parent = cur;cur = cur->_pLeft;}else if (cur->_kv.first < kv.first){parent = cur;cur = cur->_pRight;}//找到相同节点,AVL树不能插入相同节点else{return false;}}//3.插入节点//先判断是插入左边还是右边if (parent->_kv.first > kv.first){//插入左边parent->_pLeft = newnode; }else{//插入右边parent->_pRight = newnode;}newnode->_pParent = parent;cur = newnode;//4.更新平衡因子while (parent){if (parent->_pLeft == cur)parent->_bf--;elseparent->_bf++;// 更新后检测双亲的平衡因子if (parent->_bf == 0){break;}else if (parent->_bf == 1 || parent->_bf == -1){//平衡因子需要继续往上更新cur = parent;parent = parent->_pParent;}else if (parent->_bf == 2 || parent->_bf == -2){if (parent->_bf == -2 && cur->_bf == -1){//左边高进行右单旋RotateR(parent);}else if (parent->_bf == 2 && cur->_bf == 1){//右边高进行左单旋RotateL(parent);}else if (parent->_bf == 2 && cur->_bf == -1){RotateRL(parent);}else//(parent->_bf == -2 && cur->_bf == 1){RotateLR(parent);}break;}else{//其他情况,断言报错assert(false);}}return true;}
这里要注意AVL树不能插入相同节点
AVL树插入新节点的逻辑结构如上述代码所示,如果在一棵原本是平衡的AVL树中插入一个新节点,可能造成不平衡,此时必须调整树的结构,使之平衡化。根据节点插入位置的不同,AVL树的旋转分为四种:那么我们具体来看看AVL树旋转的实现:
✨左单旋
新节点插入较高右子树的右侧—右右:左单旋
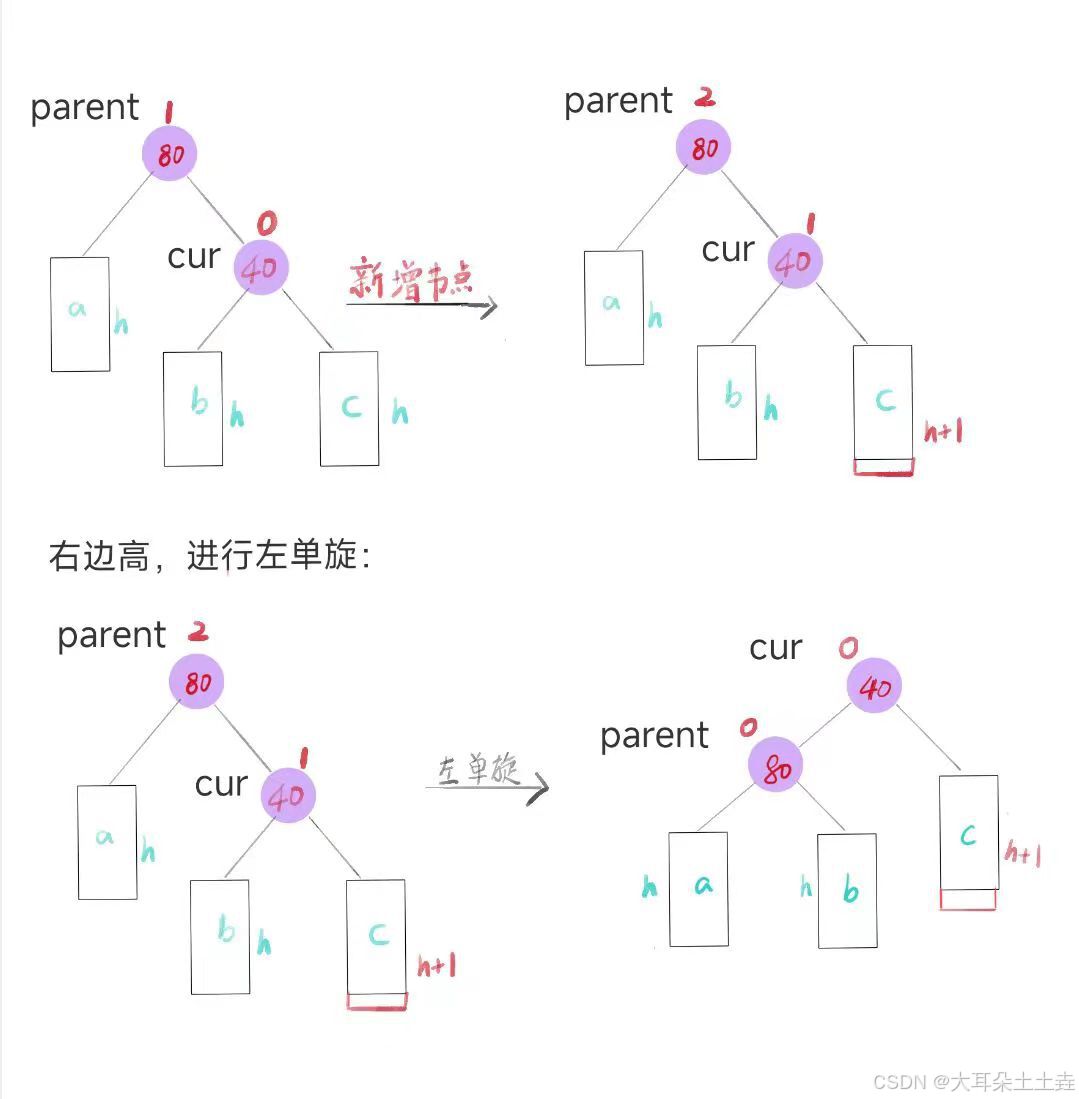
parent和cur的平衡因子经过旋转之后变为0,维持了AVL树的平衡。
代码如下:
// 左单旋
void RotateL(Node* parent)
{Node* cur = parent->_pRight;//将cur的左边给parent的右边,cur的左边再指向parentparent->_pRight = cur->_pLeft;cur->_pLeft = parent;//链接cur与parent的父节点if (parent->_pParent == nullptr){//如果pParent是根节点cur->_pParent = nullptr;_pRoot = cur;}else if (parent->_pParent->_pLeft == parent)parent->_pParent->_pLeft = cur;elseparent->_pParent->_pRight = cur;//更新父节点cur->_pParent = parent->_pParent;parent->_pParent = cur;if(parent->_pRight)//判断pParent的右边是否存在parent->_pRight->_pParent = parent;//更新平衡因子parent->_bf = 0;cur->_bf = 0;
}
✨右单旋
新节点插入较高左子树的左侧—左左:右单旋
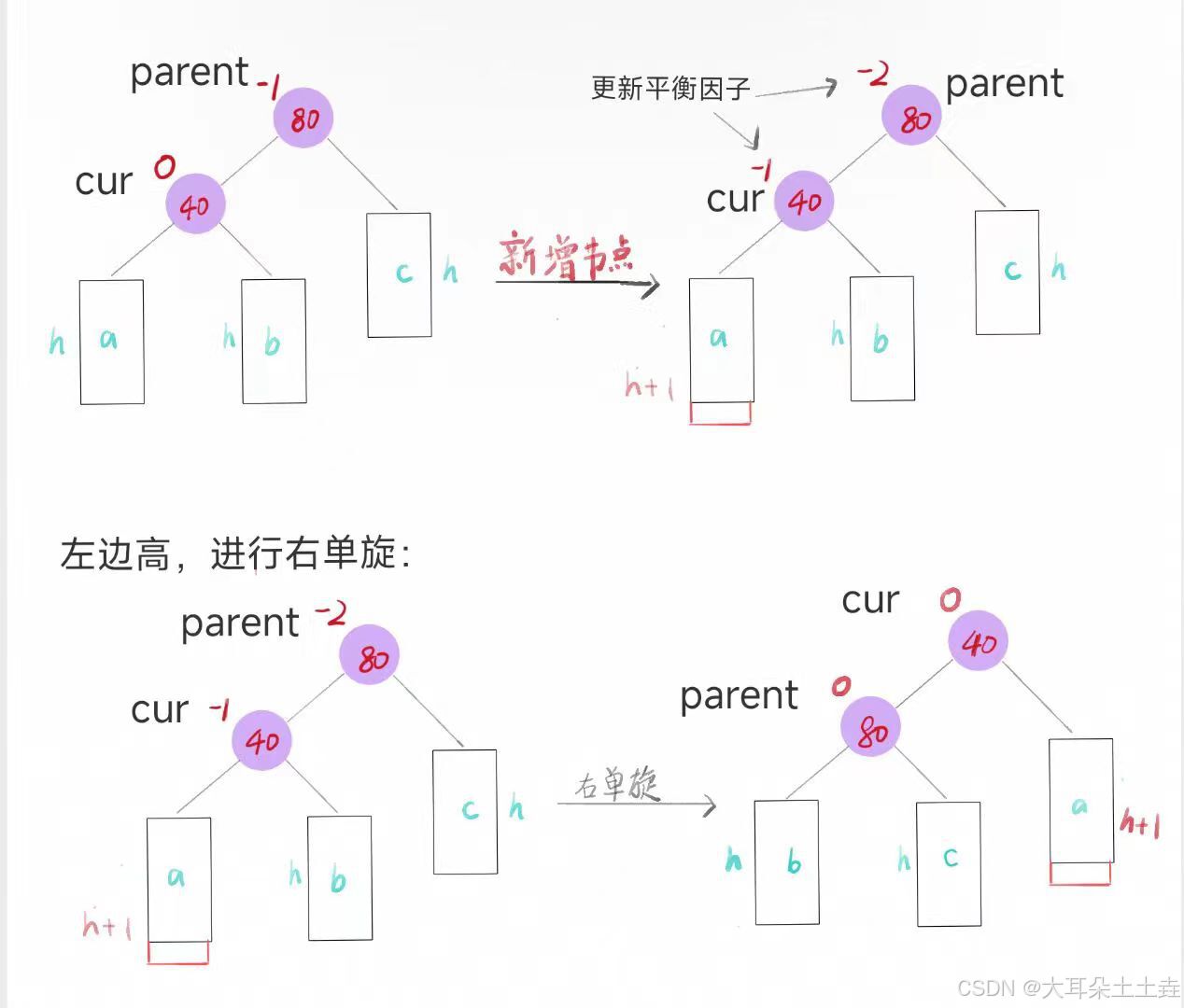
代码如下:
// 右单旋void RotateR(Node* parent){Node* cur = parent->_pLeft;//将cur的右边给pParent的左边,cur的右边再指向pParentparent->_pLeft = cur->_pRight;cur->_pRight = parent;//链接cur与pParent的父节点if (parent->_pParent == nullptr){//如果pParent是根节点cur->_pParent = nullptr;_pRoot = cur;}else if (parent->_pParent->_pLeft == parent)parent->_pParent->_pLeft = cur;elseparent->_pParent->_pRight = cur;//更新父节点cur->_pParent = parent->_pParent;parent->_pParent = cur;if (parent->_pLeft)parent->_pLeft->_pParent = parent;//更新平衡因子parent->_bf = 0;cur->_bf = 0;}
✨右左双旋
新节点插入较高右子树的左侧—右左:先右单旋再左单旋,借助上面实现的右单旋和左单旋即可
如下图所示,较高右子树(以cur节点为根节点的树)的左侧(以child节点为根节点的树),插入节点,注意这里可以插入child的左侧或右侧,只要插入在child的子树上即可,所以可以是下图中的b或c,这里选择b:
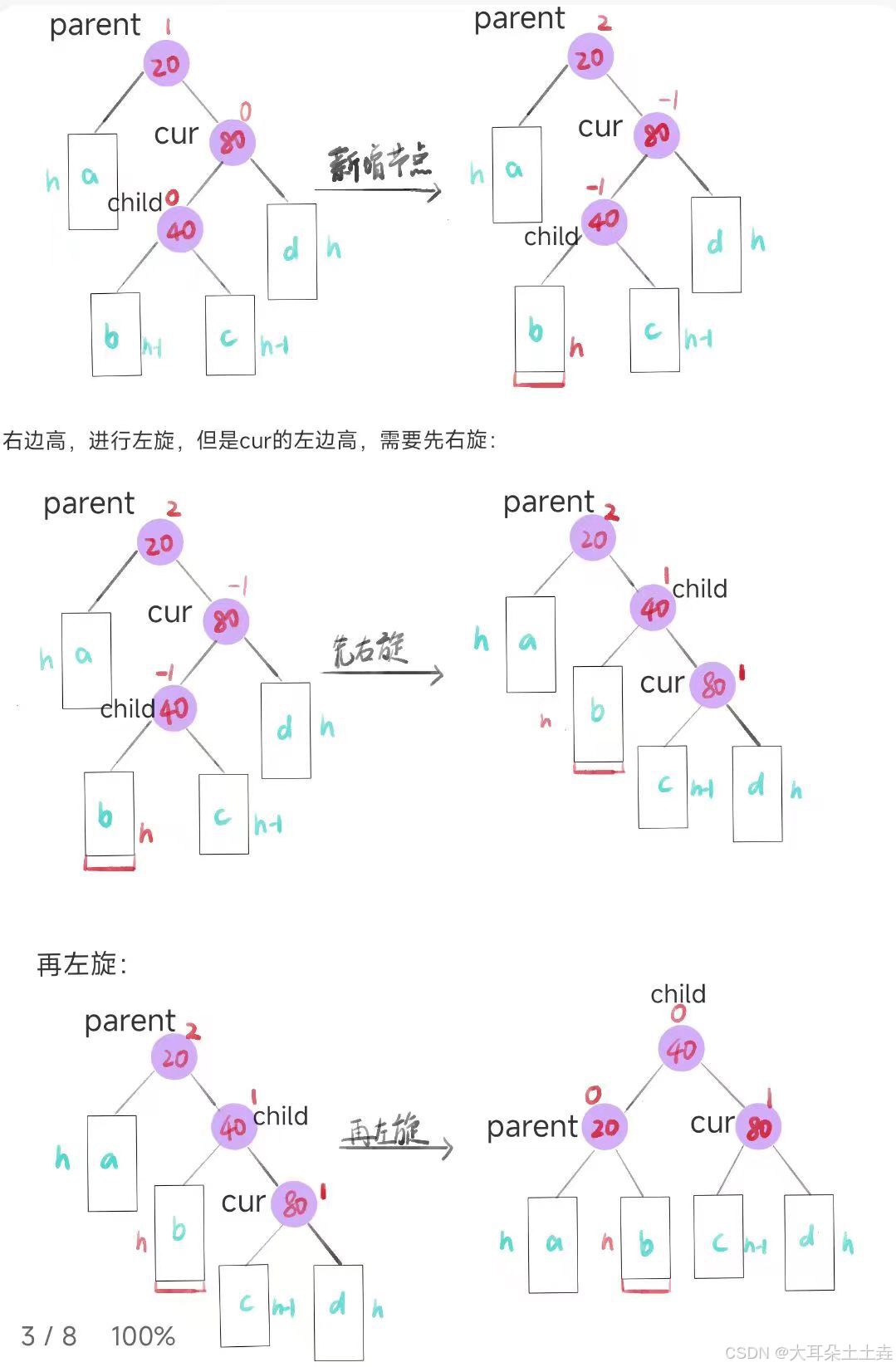
前文我们说过只要插入在child的子树上即可,所以可以是上图中的b或c,这里选择b,那么如果是c的话,还是需要进行左右双旋,与选b的区别在于平衡因子的不同,这里可以根据具体选择分析出来,所以在双旋之后记得根据不同的插入位置更新不同的平衡因子。
代码如下:
// 右左双旋
void RotateRL(Node* parent)
{Node* cur = parent->_pRight;Node* child = cur->_pLeft;//旋转前保存child的平衡因子int bf = child->_bf;//cur的左边高,先右旋RotateR(cur);//再左旋RotateL(parent);//根据不同插入位置更新不同的平衡因子if (bf == -1)//插入在b{cur->_bf = 1;}else if (bf == 1)//插入在c{parent->_bf = -1;}
}
✨左右双旋
新节点插入较高左子树的右侧—左右:先左单旋再右单旋,借助上面实现的右单旋和左单旋即可
如下图所示,左右双旋与右左双旋类似,也可以插入在下图中的b或从,旋转方式一样,不影响,就是最后平衡因子需要根据插入的位置更新:
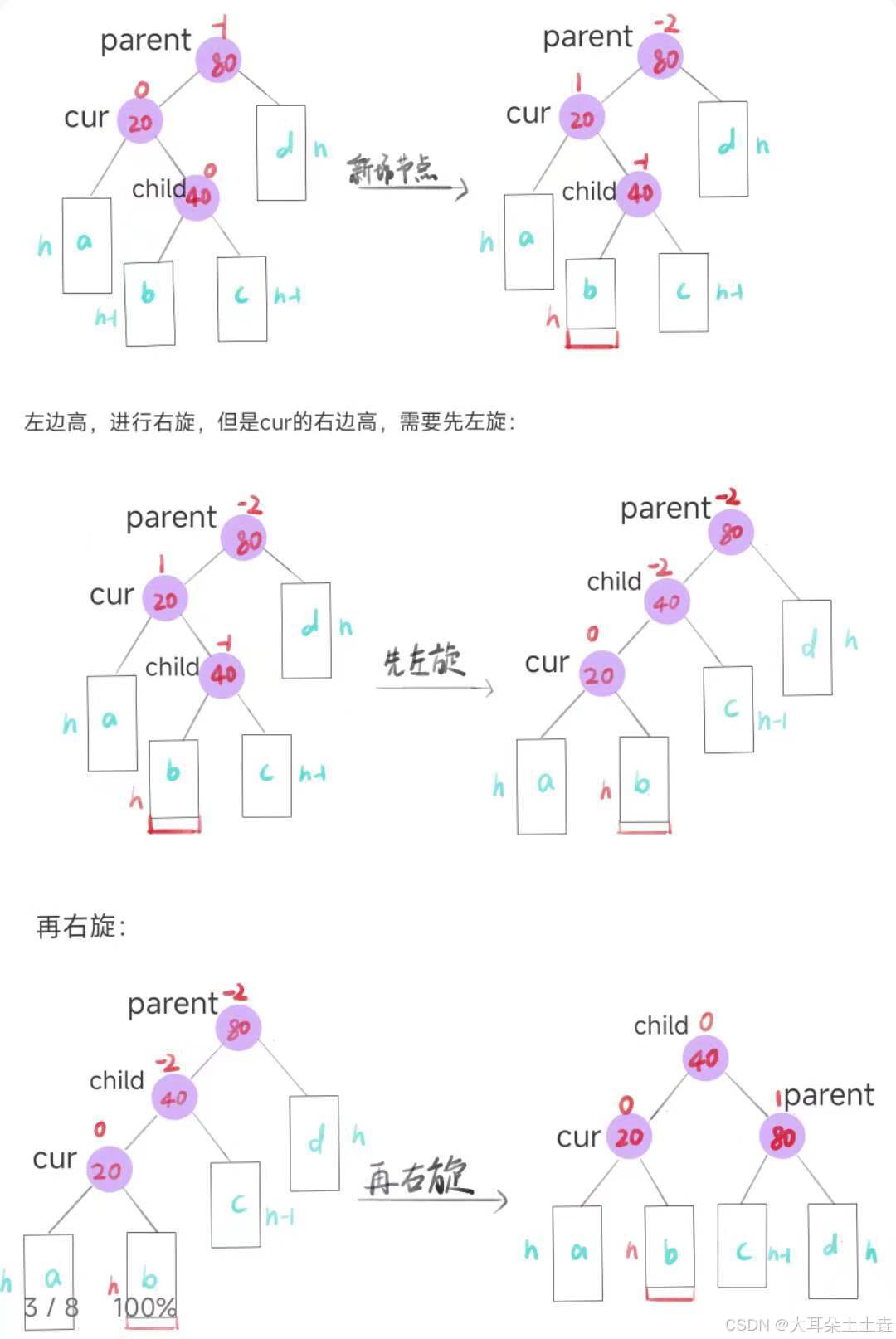
代码如下:
// 左右双旋
void RotateLR(Node* parent)
{Node* cur = parent->_pLeft;Node* child = cur->_pRight;//旋转前保存child的平衡因子int bf = child->_bf;//cur的右边高,先左旋RotateL(cur);//再右旋RotateR(parent);//根据不同插入位置更新不同的平衡因子if (bf == -1)//b{parent->_bf = 1;}else if (bf == 1)//c{cur->_bf = -1;}
}小结:
假如以parent为根的子树不平衡,即parent的平衡因子为2或者-2,分以下情况考虑
- parent的平衡因子为2,说明parent的右子树高,设parent的右子树的根为cur
当cur的平衡因子为1时,执行左单旋
当cur的平衡因子为-1时,执行右左双旋 - parent的平衡因子为-2,说明parent的左子树高,设parent的左子树的根为cur
当cur的平衡因子为-1是,执行右单旋
当cur的平衡因子为1时,执行左右双旋
旋转完成后,原parent为根的子树个高度降低,已经平衡,不需要再向上更新。
3.中序遍历
AVL树就是在二叉搜索树的基础上引入了平衡因子,因此AVL树也可以看成是二叉搜索树,其中序遍历和我们之前实现过的二叉搜索树一样。
代码如下:
//中序遍历
void InOrder()
{_InOrder(_pRoot);
}void _InOrder(Node* root)
{if (root == nullptr){return;}_InOrder(root->_pLeft);cout << root->_kv.first << ":" << root->_kv.second << endl;_InOrder(root->_pRight);}
4. AVL树的验证
AVL树是在二叉搜索树的基础上加入了平衡性的限制,因此要验证AVL树,可以分两步:
- 验证其是否为二叉搜索树:
如果中序遍历可得到一个有序的序列,就说明为二叉搜索树 - 验证其是否为平衡树:
每个节点子树高度差的绝对值不超过1
对于验证是否是平衡树,代码如下:
bool IsBalanceTree()
{
//嵌套一层函数return _IsBalanceTree(_pRoot);
}
bool _IsBalanceTree(Node* pRoot)
{// 空树也是AVL树if (nullptr == pRoot) return true;// 计算pRoot节点的平衡因子:即pRoot左右子树的高度差int leftHeight = _Height(pRoot->_pLeft);int rightHeight = _Height(pRoot->_pRight);int diff = rightHeight - leftHeight;// 如果计算出的平衡因子与pRoot的平衡因子不相等,或者pRoot平衡因子的绝对值超过1,则一定不是AVL树if (diff != pRoot->_bf || (diff > 1 || diff < -1))return false;// pRoot的左和右如果都是AVL树,则该树一定是AVL树return _IsBalanceTree(pRoot->_pLeft) && _IsBalanceTree(pRoot -> _pRight);
}//求树的高度
size_t _Height(Node* pRoot)
{if (pRoot == nullptr)return 0;size_t left = _Height(pRoot->_pLeft);size_t right = _Height(pRoot->_pRight);return (left >= right ? left : right) + 1;
}
计算pRoot节点的平衡因子:即计算pRoot左右子树的高度差,我们利用递归实现即可。计算是否为平衡树因为是递归需要传递根节点,但是我们在使用时并不能获取根节点,所以需要嵌套一层函数。
5.验证用例
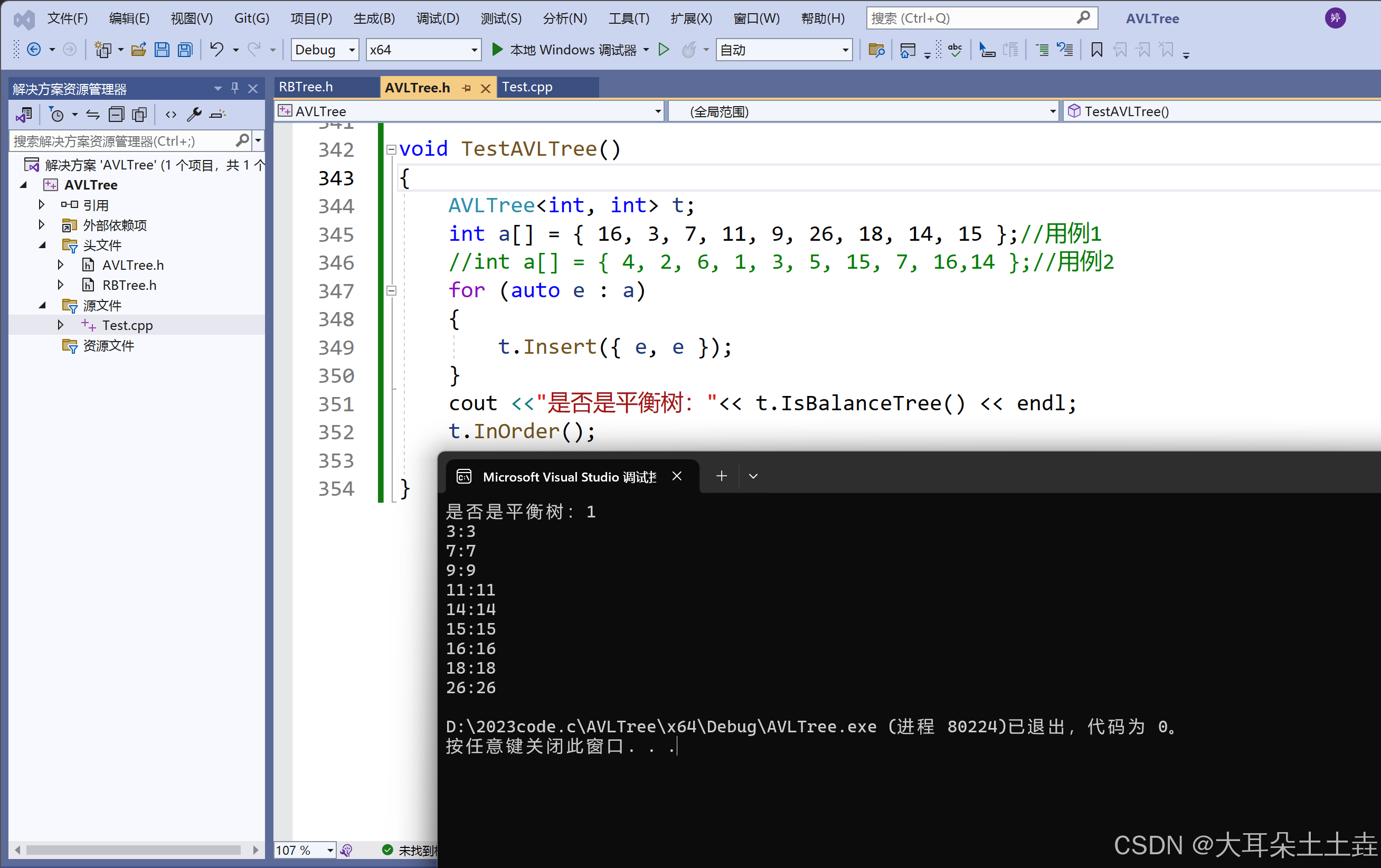
void TestAVLTree()
{AVLTree<int, int> t;int a[] = { 16, 3, 7, 11, 9, 26, 18, 14, 15 };//用例1//int a[] = { 4, 2, 6, 1, 3, 5, 15, 7, 16,14 };//用例2for (auto e : a){t.Insert({ e, e });}cout <<"是否是平衡树:"<< t.IsBalanceTree() << endl;t.InOrder();}
结果如下:
6.结语
因为AVL树也是二叉搜索树,其他的类似查找节点,析构函数和构造函数都与二叉搜索树类似,对于删除节点,可按照二叉搜索树的方式将节点删除,然后再更新平衡因子,最差情况下一直要调整到根节点的位置,大家有兴趣可以自己查找了解一下,以上就是今天所有的内容啦~ 完结撒花 ~🥳🎉🎉














![【扒代码】X = output[:,:,y1:y2,x1:x2].sum()](http://pic.xiahunao.cn/【扒代码】X = output[:,:,y1:y2,x1:x2].sum())




